— manufactured in Miraj (read paper)
This article demonstrates the theoretical and practical construction of microtonal scales for the intonation of North Indian ragas, using tools available with the Bol Processor (BP3) + Csound.
It is intended to complement the pages Microtonality and Just intonation, a general framework and The Two-vina experiment. However, its understanding does not require a prior study of these related pages.
This raga intonation exercise demonstrates BP3’s ability to handle sophisticated models of micro-intonation and to support the fruitful creation of music embodying these models.
Theory versus practice
To summarise the background, the framework for constructing ‘just intonation’ scales is a deciphering of the first six chapters of the Nāṭyaśāstra, a Sanskrit treatise on music, dance and drama dating from a period between 400 BC and 200 AD. For convenience, we call it “Bharata’s Model”, although there is no historical record of a single author by that name.
Using exclusive information driven from the text and its description of the Two-vina experiment, an infinite number of valid interpretations of the ancient theory are possible, as shown in A Mathematical Discussion of the Ancient Theory of Scales according to Natyashastra (Bel 1988a). Among these, the one advocated by many musicologists — influenced by western acoustics and scale theories — is that the frequency ratio of the harmonic major third would be 5/4. This is equivalent to setting the frequency ratio of the syntonic comma at 81/80.
Although this interpretation provides a consistent model for just intonation harmony - see Just intonation, a general framework — it would be a stretch to claim that the same applies to raga intonation. Accurate assessment of raga performance using our Melodic Movement Analyser (MMA) in the early 1980s revealed that melodic structures derived from statistics (using selective tonagrams, see below) often differ significantly from the scales predicted by the “just intonation” interpretation of Bharata’s model. Part of the explanation may be the strong harmonic attraction of drones (tanpura) played in the background of raga performances.
Speaking of grama-s (scale frameworks) in the ancient Indian theory, E.J. Arnold wrote (1982 p. 40):
Strictly speaking the gramas belong to that aspect of nada (vibration) which is anahata (“unstruck”). That means to say that the “grama” can never be heard as a musical scale [as we did on page Just intonation, a general framework]. What can be heard as a musical scale is not the grama, but any of its murcchanas.
Once electronic devices such as the Shruti Harmonium (1979) and the Melodic Movement Analyser (1981) became available, the challenge for raga intonation research was to reconcile two methodologies: a top-down approach, testing hypothetical models against data, and a data-driven bottom-up approach.
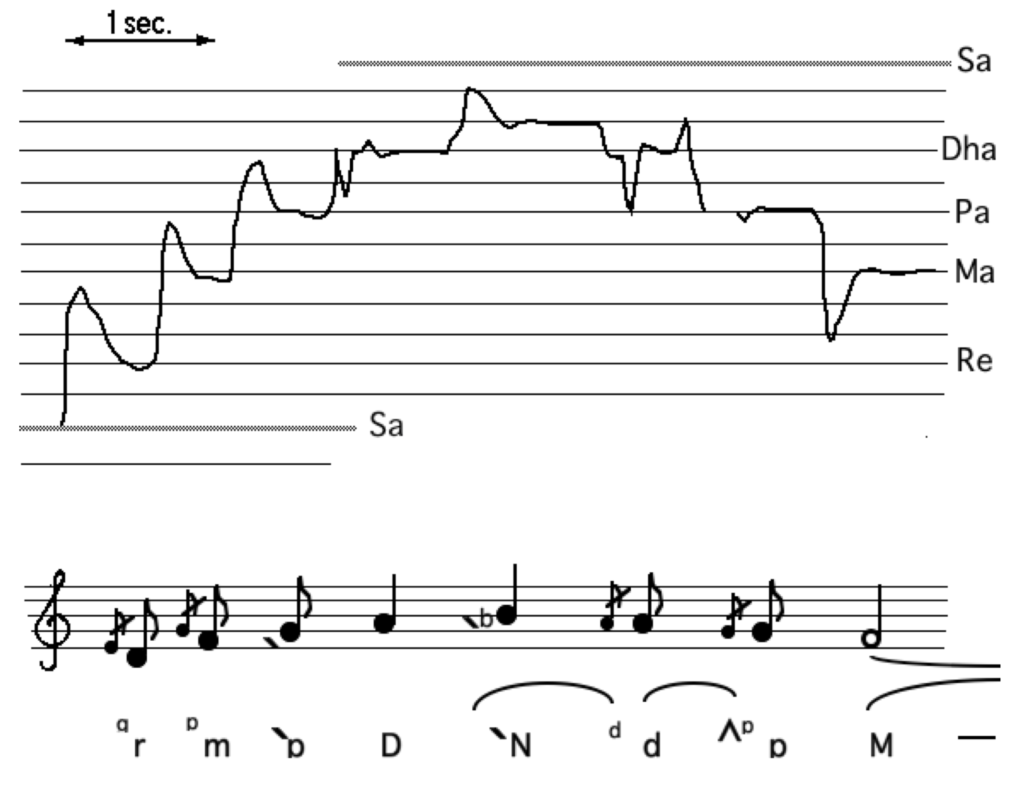
The “microscopic” observation of melodic lines (now easily rendered by software such as Praat) has confirmed the importance of note treatment (ornamentation, alankara) and temporal dimensions of raga that are not taken into account by scale theories. For example, the rendering of the note ‘Ga’ in raga Darbari Kanada (Bel & Bor 1984; van der Meer 2019) and the typical treatment of notes in other ragas (e.g. Rao & Van der Meer 2009; 2010) have been discussed at length. The visual transcription of a phrase from raga Asha illustrates this:
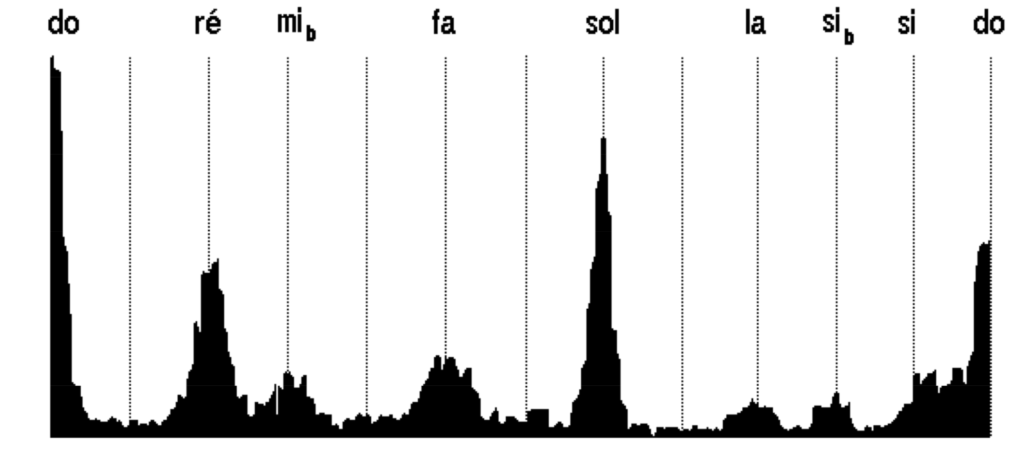
To extract scale information from this melodic continuum, a statistical model was implemented to show the distribution of pitch over an octave. The image shows the tonagram of a 2-minute sketch (chalana) of raga Sindhura taught by Pandit Dilip Chandra Vedi.
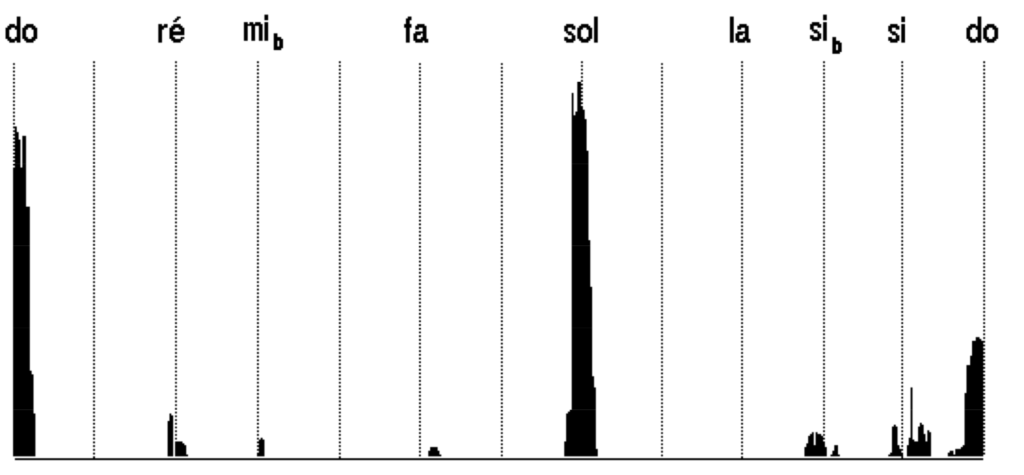
The same melodic data was processed again after filtering through 3 windows that attempted to isolate ‘stable’ parts of the line. The first window, typically 0.1 seconds, would eliminate irregular segments, the second (0.4 seconds) would discard segments outside a rectangle of 80 cents height, and the third was used for averaging. The result is a “skeleton” of the tonal scale, displayed as a selective tonagram.
These results would often not match the scale metrics predicted by the ‘just intonation’ interpretation of Bharata’s model. Continuing with this data-driven approach, we produced the (non-selective) tonagrams of 30 ragas (again, chalana-s) to compute a classification based on their tonal material. Dissimilarities between pairs of graphs (computed using Kuiper’s algorithm) were approximated as distances, from which a 3-dimensional classical scaling was extracted:
This experiment suggests that contemporary North-Indian ragas are amenable to meaningful automatic classification on the basis of their (time-independent) intervalic content alone. This approach is analogous to human face recognition techniques, which are able to identify related images from a limited set of features.

at the National Centre for the Performing Arts (Mumbai) in 1983
This impressive classification has been obtained by statistical analysis of static representations of raga performance. This means that the same result would be obtained by playing the sound file in reverse, or even by slicing it into segments reassembled in a random order… Music is a dynamic phenomenon that cannot be reduced to tonal “intervals”. Therefore, subsequent research into the representation of the melodic lines of raga — once it could be efficiently processed by 100% digital computing — led to the concept of Music in Motion, i.e. synchronising graphs with sounds so that the visuals reflect the music as it is being heard, arguably the only appropriate“notation” for raga (Van der Meer & Rao 2010; Van der Meer 2020).
This graph model is probably a great achievement as an educational and documentary tool, indeed the environment I dreamed of when designing the Melodic Movement Analyser. However, to promote it as a theoretical model is the continuation of a western selective bias. As far as I know, no Indian music master has ever attempted to describe the intricacies of raga using hand-drawn melograms, although they could. The fascination with technology — and western ‘science’ in general — is no indication of its relevance to ancient Indian concepts.
Music is judged by ears; therefore, a theory of music should be judged by its ability to produce musical sounds via predictive model(s). Numbers, charts and graphs are merely tools for interpreting and predicting sound phenomena. This approach is called analysis by synthesis in Daniel Hirst’s book on speech prosody. (Hirst, 2022, forthcoming, p. 137):
Analysis by synthesis involves trying to set up an explicit predictive model to account for the data which we wish to describe. A model, in this sense, is a system which can be used for analysis — that is deriving a (simple) abstract underlying representation from the (complicated) raw acoustic data. A model which can do this is explicit but it is not necessarily predictive and empirically testable. To meet these additional criteria, the model must also be reversible, that is it must be possible to use the model to synthesise observable data from the underlying representation.
This is the raison d’être for the following investigation.
Microtonal framework
The “flexible” model derived from the theoretical model of Natya Shastra (see The Two-vina experiment) rejects the claim of a precise frequency ratio for the harmonic major third classified in ancient literature as anuvadi (asonant). This amounts to admitting that the syntonic comma (pramāņa ṣruti in Sanskrit) could take any value between 0 and 56.8 cents.
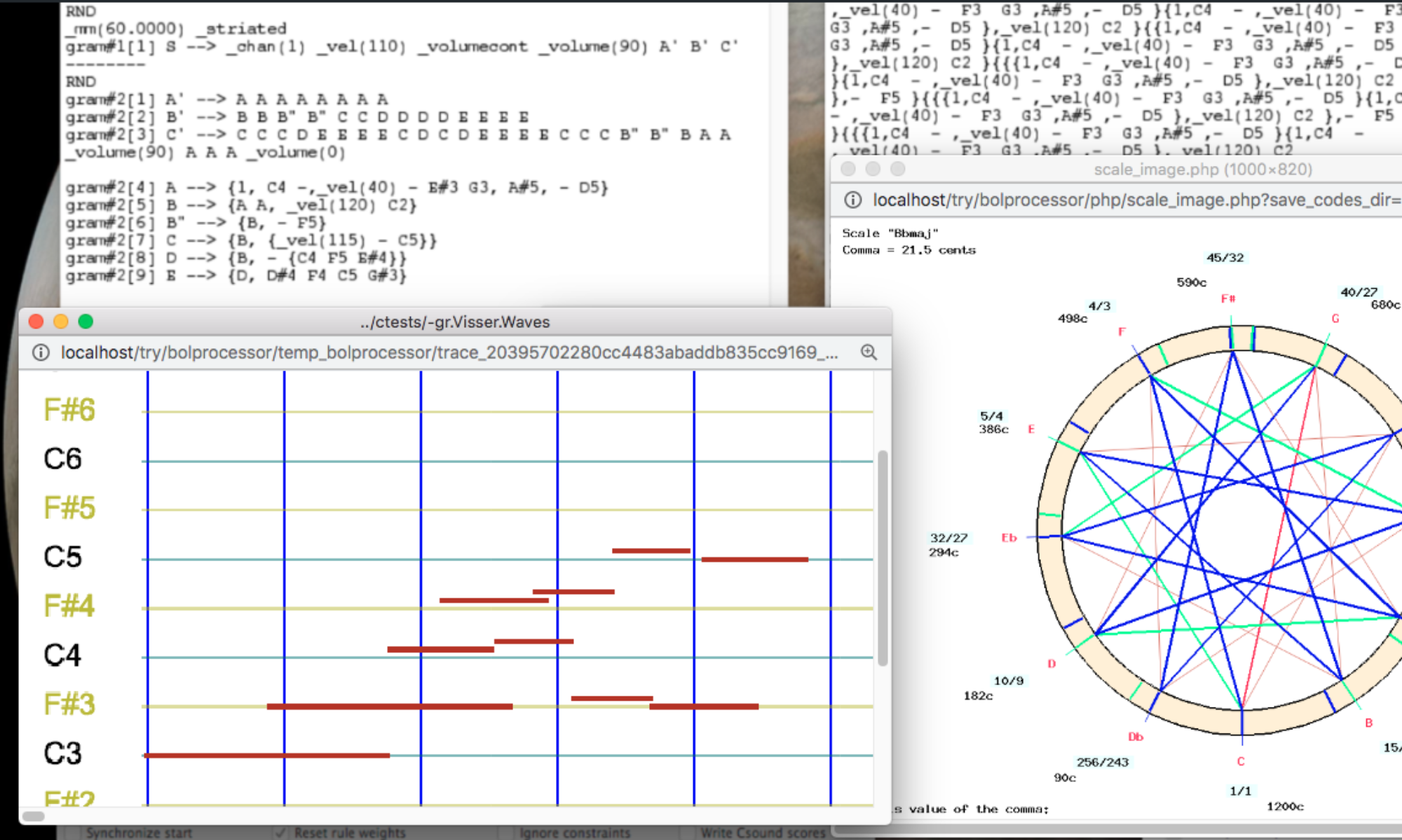
Let us look at some graphical representations (from the Bol processor) to illustrate these points.
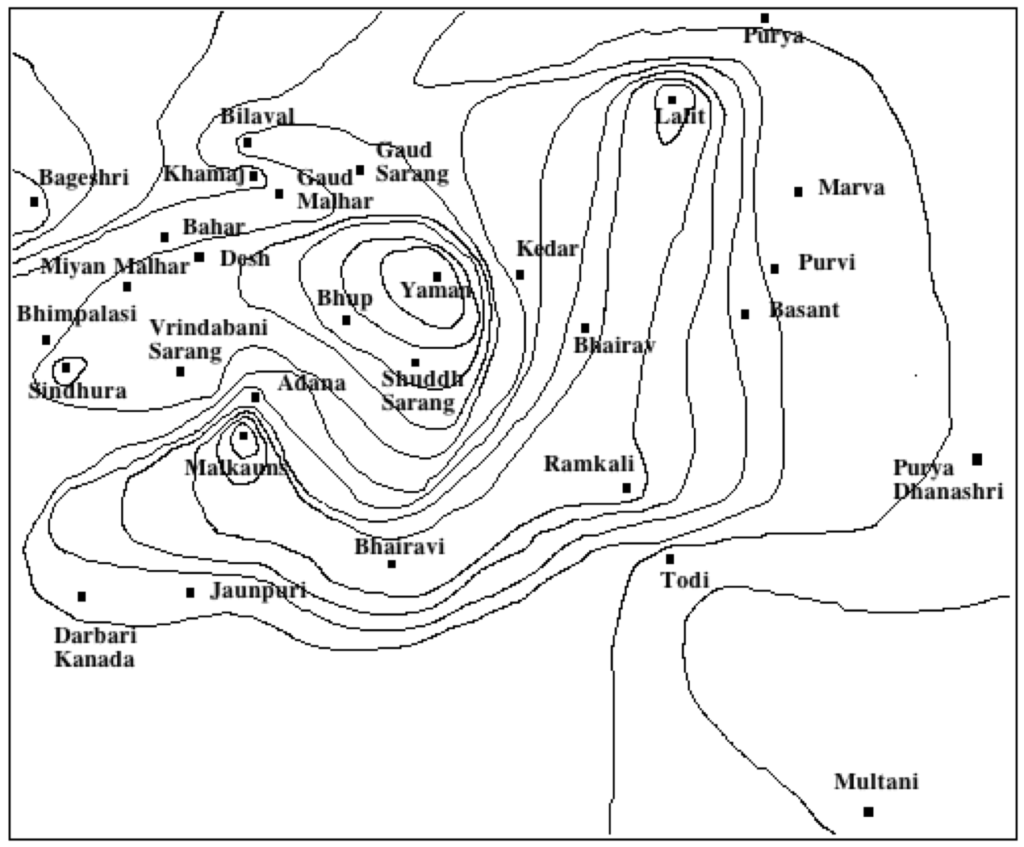
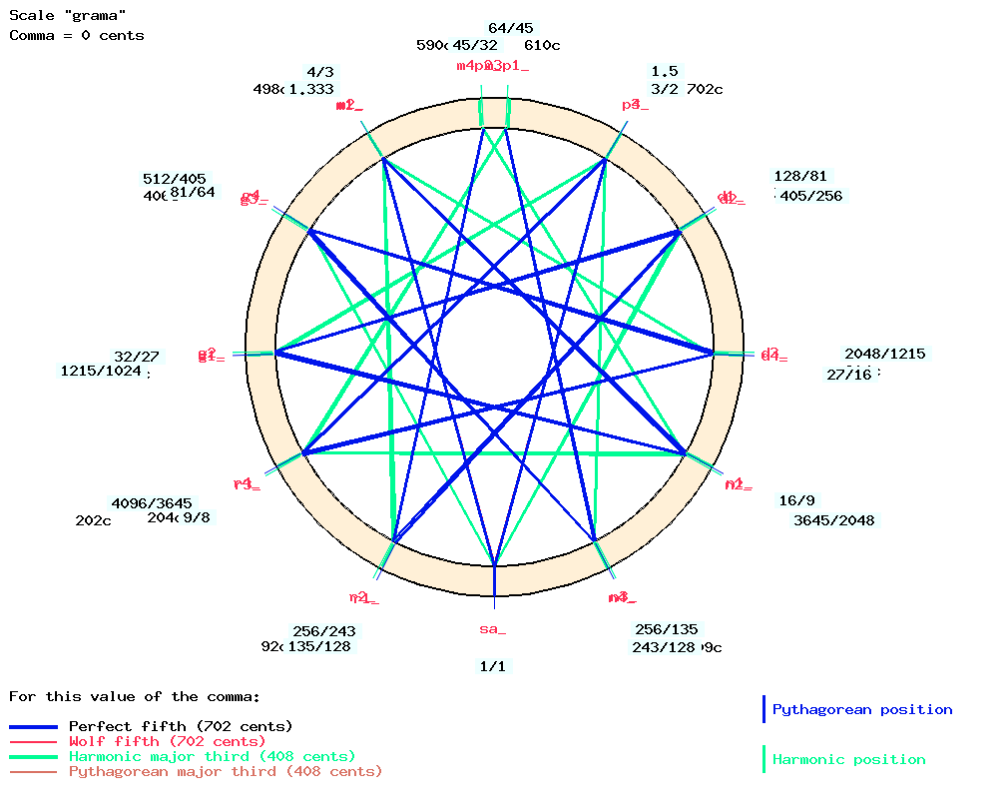
The basic framework of musical scales, according to Indian musicology, is a set of 22 tonal positions in the octave called shruti-s in ancient texts. Below is the framework displayed by the Bol Processor (microtonal scale “grama”) with a 81/80 syntonic comma. The names of the positions “r1_”, “r2_”, etc. follow the constraints of lower case initials and the addition of an underscore to distinguish octave numbers. Positions “r1” and “r2” are two ways of locating komal Re (“Db” or “re bemol”), while “r3” and “r4” denote shuddha Re (“D” or “re”), etc.
These 22 shruti-s can be heard on the page Just intonation, a general framework, bearing in mind (see above) that this is a framework and not a scale. No musician would ever attempt to play or sing these positions as “notes”!
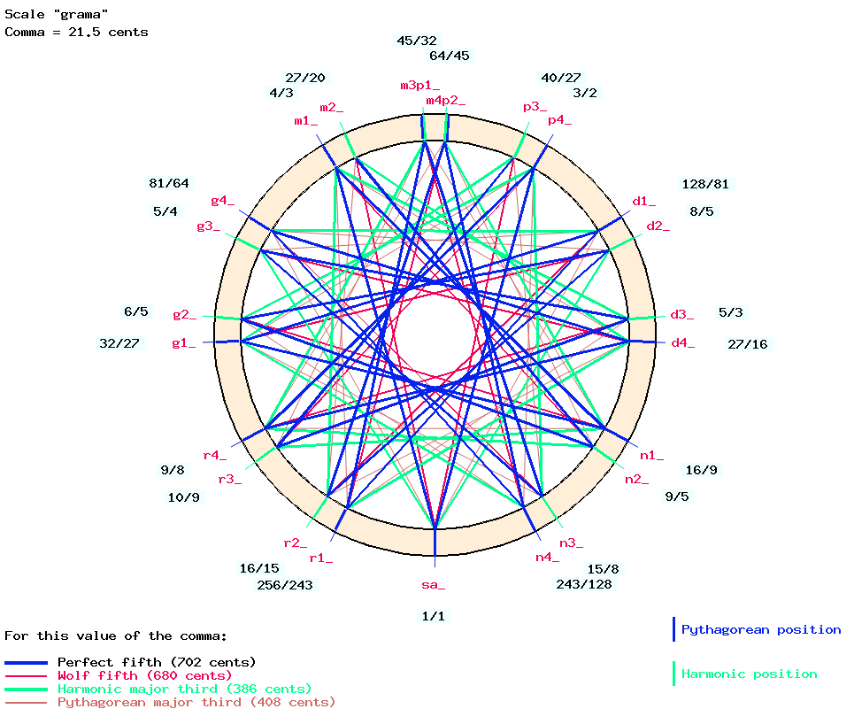
What happens if the value of the syntonic comma is changed? Below is the same framework with a comma of 0 cent. In this case, any “harmonic position” — one whose fraction contains a multiple of 5 — moves to its nearest Pythagorean neighbour (only multiples of 3 and 2). The result is a “Pythagorean tuning”. At the top of the circle, the remaining gap is a Pythagorean comma. The positions are slightly blurred because of the mismatches associated with a very small interval (the schisma).
Below is the framework with a syntonic comma of 56.8 cents (its upper limit):
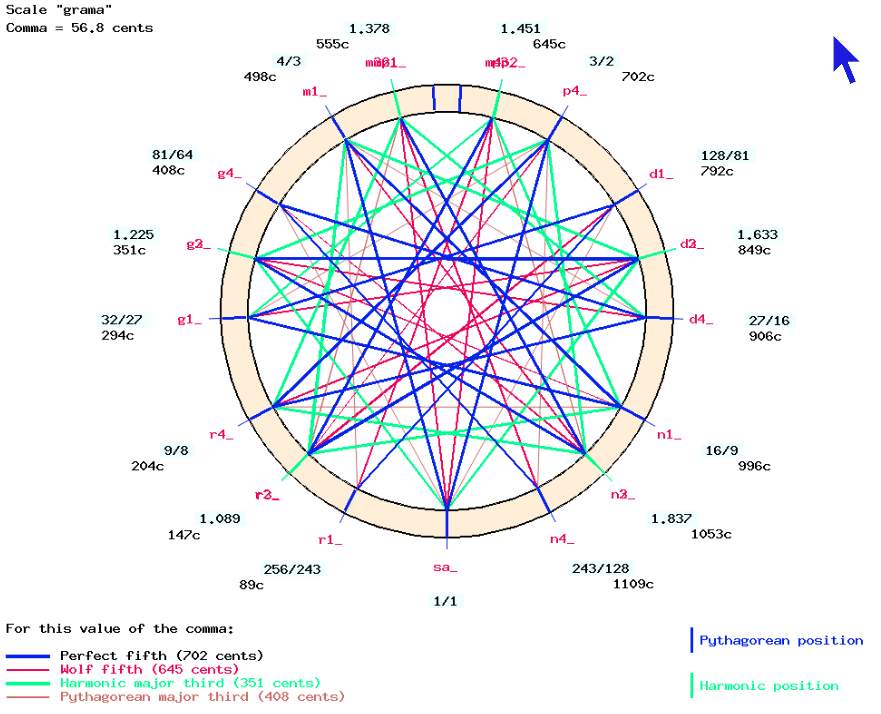
In this representation, “harmonic major thirds” of 351 cents would most likely sound “out of tune” because the 5/4 ratio yields 384 cents. In fact, “g2” and “g3” are both distant by a quarter tone between Pythagorean “g1” (32/27) and Pythagorean “g4” (81/64). Nevertheless, the internal consistency of this framework (counting perfect fifths in blue) makes it suitable for constructing musical scales.
Between these limits of 0 and 56.8 cents, the graphic representation of the scales and their internal tonal structure remain unchanged, bearing in mind that the size of the major-third intervals is determined by the syntonic comma.
Construction of scale types

The model taken from Bharata’s Natya Shastra is not an obvious reference for prescribing raga intonation, as this musical genre came into existence a few centuries later.
Most of the background knowledge required for the following presentation is borrowed from Bose (1960) and my late colleague E. James Arnold who published A Mathematical model of the Shruti-Swara-Grama-Murcchana-Jati System (Journal of the Sangit Natak Akademi, New Delhi 1982). Arnold studied Indian music in Banaras and Delhi in the 1970s and the early 1980s.
Bose was convinced (1960 p. 211) that the scale called Kaishika Madhyama was equivalent to a “just-intonation” seven-degree scale of western musicology. In other words, he took it for granted that the 5/4 frequency ratio (harmonic major third) should be equivalent to the 7-shruti interval, but this statement had no influence on the rest of his analysis.

Arnold (1982 p. 17) immediately used integer ratios to construct intervals with the fixed syntonic comma (81/80), but, as suggested above, this does not affect his model in terms of its structural description. He insisted on setting up a “geometric model” rather than a speculative description based on numbers, as many authors (e.g. Alain Daniélou) had attempted. The most innovative aspect of Arnold’s study was the use of a circular sliding model to illustrate the matching of intervals in transposition processes (murcchana-s) - see page The Two-vina experiment.
In reality, it would be more convenient to continue to express all intervals in numbers of shruti-s, in accordance with the ancient Indian theory, but a machine needs metric data to draw graphics of scales. For this reason, we show graphs with a syntonic comma of 81/80, keeping in mind the possibility of changing this value later.
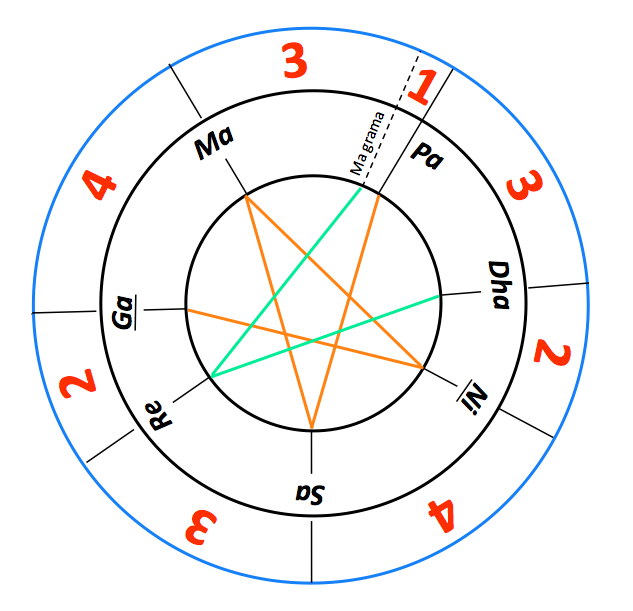
The 22-shruti framework offers the possibility of constructing 211 = 2048 chromatic scales, of which only 12 are “optimally consonant”, i.e. contain only one wolf fifth (smaller by 1 syntonic comma = 22 cents).
The building blocks of the tonal system according to traditional Indian musicology are two seven-degree scales called Ma-grama and Sa-grama. Bose (1960 p. 13) writes: the Shadja Grāma developed from the ancient tetrachord in which the hymns of the Sāma Veda were chanted. Later on another scale, called the Madhyama Grāma, was added to the secular musical system. The two scales (Dorian modes, in western terminology) differ in the position of Pa (“G” or “sol”) which may differ by a syntonic comma (pramāņa ṣruti). In the Sa-grama, the interval Sa-Pa is a perfect fifth (13 shruti-s) whereas in the Ma-grama it is a wolf fifth (12 shruti-s). Conversely, the interval Pa-Re is a perfect fifth in Ma-grama and a wolf fifth in Sa-grama.
Bharata used the Sa-grama to expose his thought experiment (The Two vinas) aimed at determining the sizes of shruti-s. He then introduced two additional notes: kakali Nishada (komal Ni or “Bflat”) and antara Gandhara (shuddh Ga or “E”) to obtain a nine-degree scale from which “optimally consonant” chromatic scales could be derived from modal transpositions (murcchana). The process of constructing these 12 chromatic scales, namely “Ma01″, “Ma02″… “Sa01″, “Sa20″, etc., is explained on the page Just intonation, a general framework.
The selection of notes in each chromatic scale produces 5 to 7 note melodic types. In Natya Shastra these melodic types are called jāti. These can be seen as the ancestors of ragas, although their lineages and structures are only speculative (read on). The term thāṭ (pronounced ‘taat’) — translated as ‘mode’ or ‘parent scale’ — was later adopted, each thāṭ being called by the name of a raga (see Wikipedia). Details of the process, terminology and surveys of subsequent musicological literature can be found in publications by Bose and other scholars.
The construction of the basic scale types is explained by Arnold (1982 p. 37-38). The starting point is the chromatic Ma-grama in its basic position — namely “Sa_murcchana” in the “-cs.12_scales” Csound resource file. This scale can be visualised, using Arnold’s sliding model, by placing the S note of the inner wheel on the S of the outer crown :
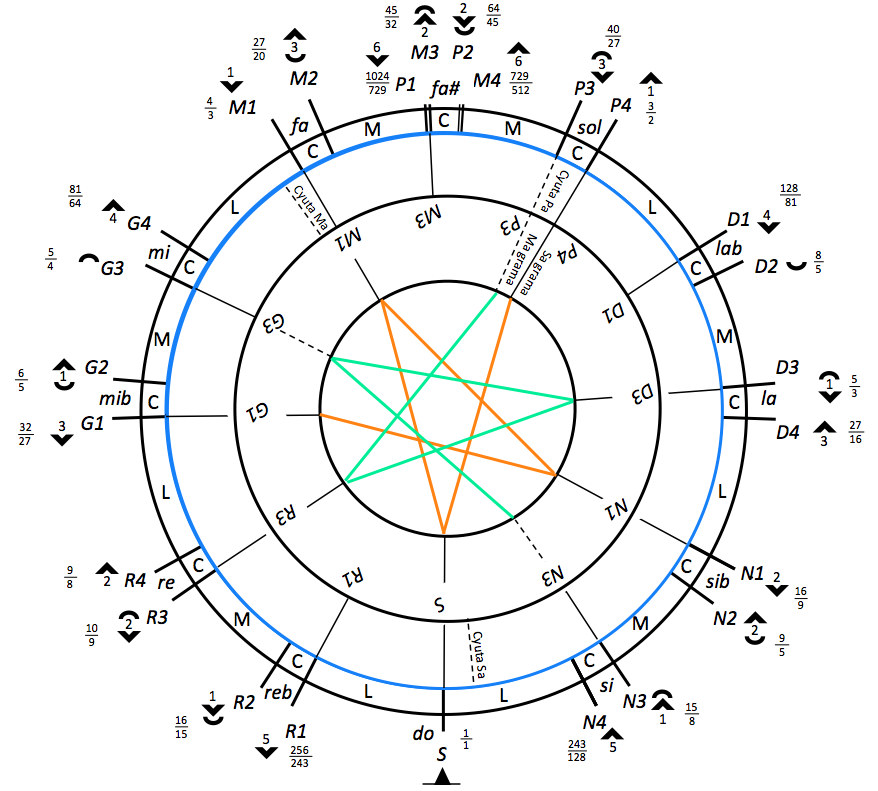
This yields the following intervals:
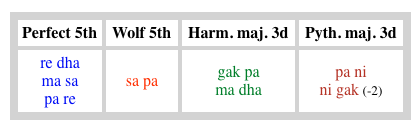
“Optimal consonance” is illustrated by two features: 1) there is only one wolf fifth (red line) in the scale (between D and G), and 2) each note is connected to every other note by a perfect fifth (blue line). This consonance is of paramount importance to Indian musicians. Consonant intervals are casually placed in melodic phrases to enhance the “flavour” of their notes, and there should be no wolf fifth in the scale.
Note that the Ma-grama chromatic scale has all its notes in their lower enharmonic positions.
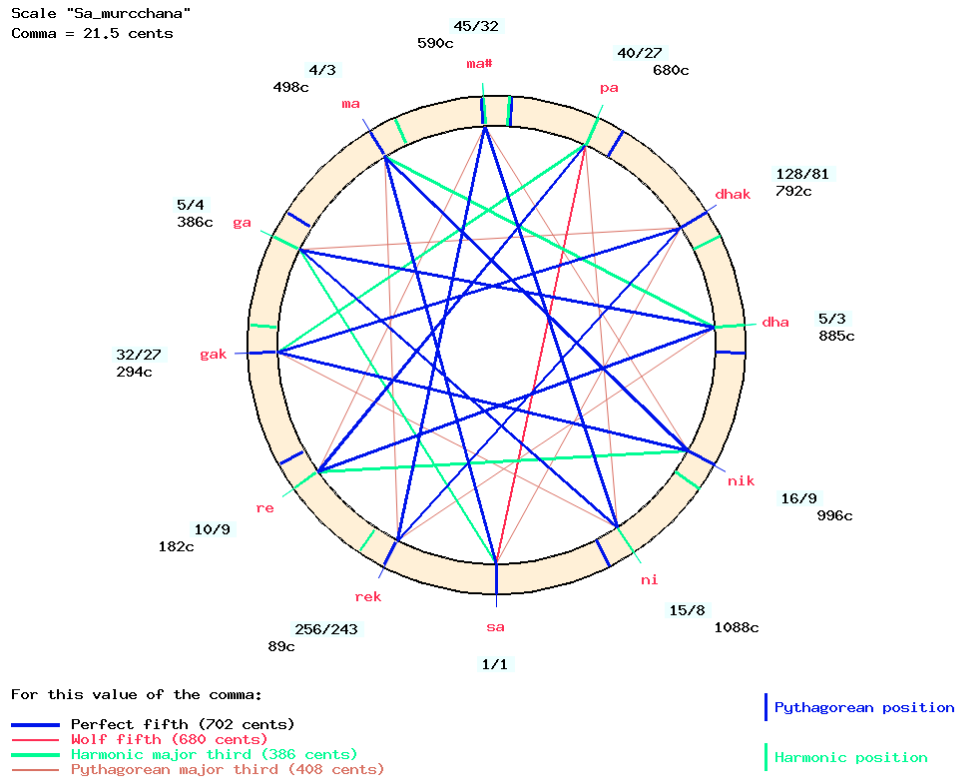
The Ma-grama chromatic scale has been renamed “Sa_murcchana” here, because ‘S’ of the moving wheel is opposite the ‘S’ of the fixed crown. The note names have been converted (with a single click) to the Indian convention. Note that the key numbers have also been (automatically) fixed to match the exclusively labelled notes. In this way, the upper “sa” is assigned key 72 instead of 83 in the “Ma01″ scale shown on the Just intonation, a general framework page. The tonal content of this “Sa_murchana” is shown in this table:
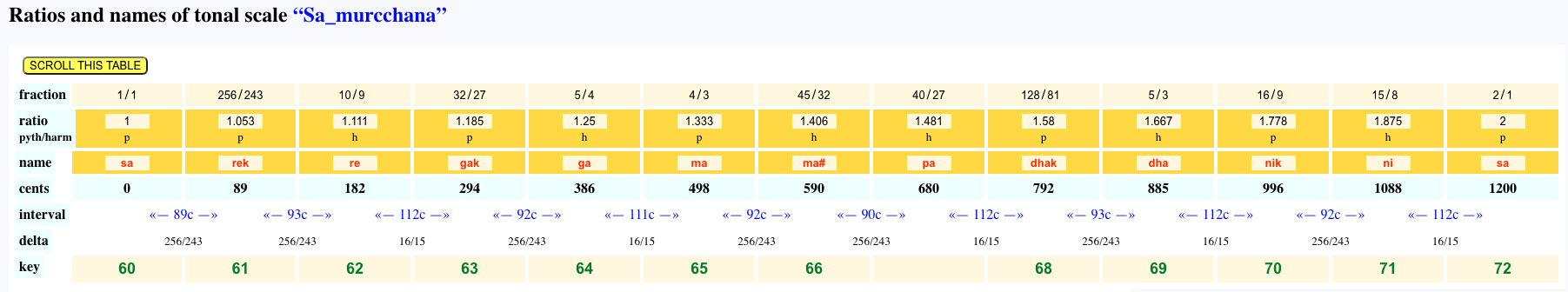
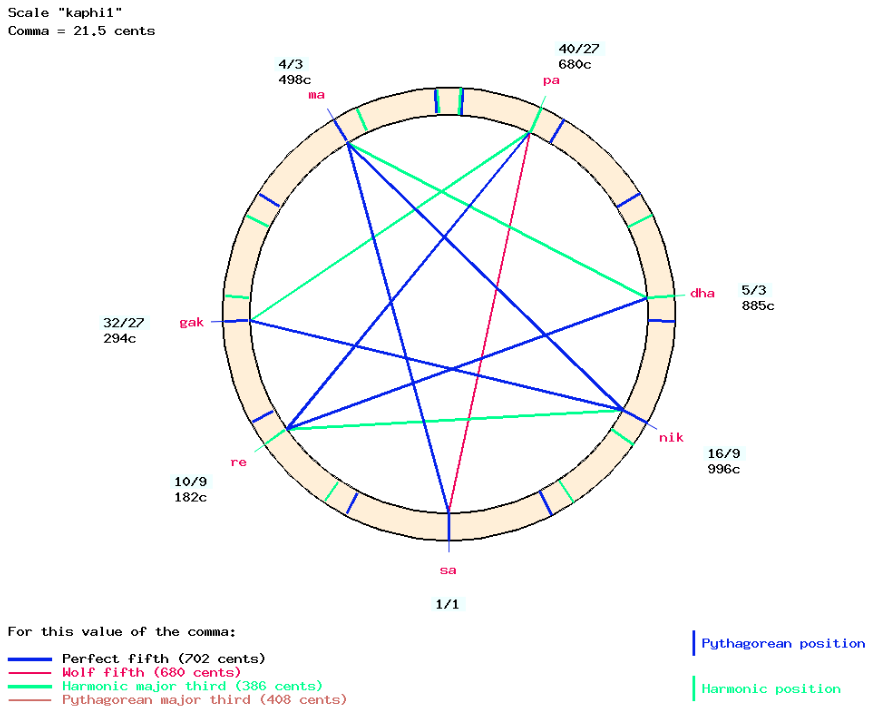
Selecting only “unaltered” notes in “Sa_murcchana” — sa, re, gak, ma, pa, dha, nik — results in the “kaphi1″ scale type named after the raga Kaphi (pronounced ‘kafi’). This can be associated with a D-mode (Dorian) in western musicology.
This scale type is saved under the name “kaphi1″ because there will be another version of the Kaphi scale type.
In “Sa_murcchana” the selection of notes can be done in two different ways:
- Select antara Gandhara (namely “ga”) in place of the scale’s Gandhara (namely “gak”), thereby raising it by 2 shruti-s. This will result in a vikrit (modified) scale type, namely “khamaj1″, associated with raga Khamaj.
- Select both antara Gandhara and kakali Nishada (namely “ni” in place of “nik” raised by 2 shruti-s) which creates the “bilaval1″ scale type associated with raga Bilaval.
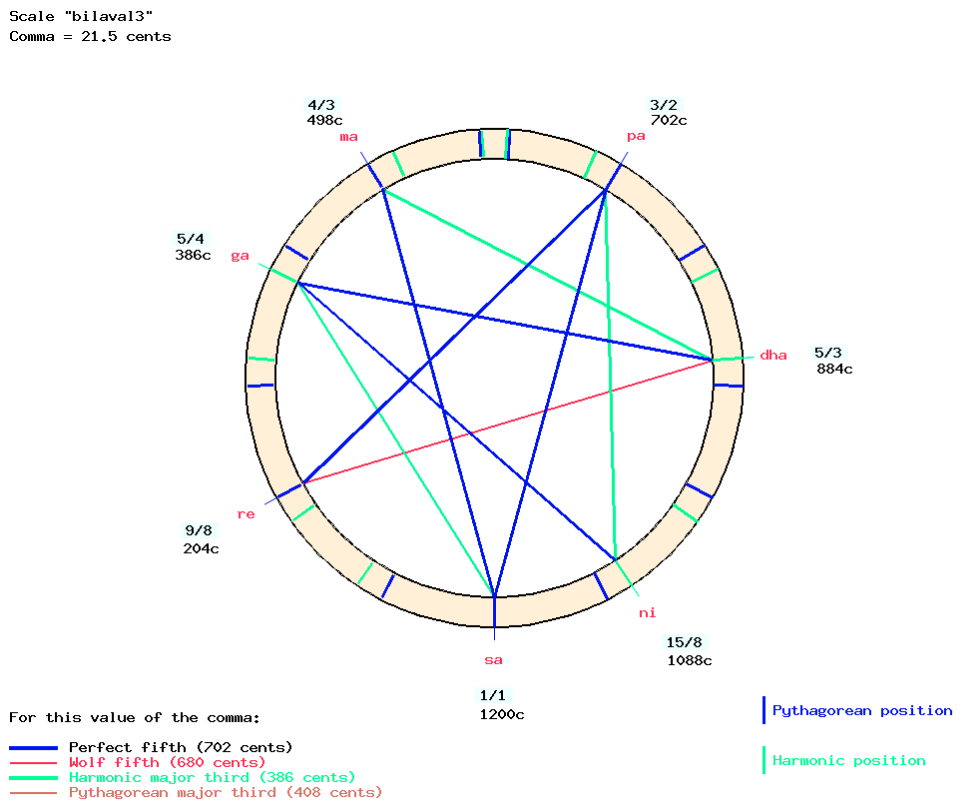
This “bilaval1″ scale type is one of three versions of the Bilaval created by the murcchana process. Although it corresponds to the scale of the white keys on a keyboard instrument, it is not the usual “just intonation” diatonic scale, because of a wolf fifth between “sa” and “pa”.
An alternative Bilaval scale type called “bilaval3″ (extracted from “Ni1_murcchana”, see below) corresponds to Giozeffo Zarlino’s “natural” scale — see Just intonation: a general framework. This is not to be confused with Zarlino’s meantone temperament discussed on the Microtonality page.
A fourth option: raising “nik” to “ni” and keeping “gak”, would produce a scale type in which “ni” has no consonant relationship with any other note in the scale. This option is therefore discarded from the model.
Each murcchana of the Ma-grama chromatic scale produces at least three types of scale by selecting unaltered notes, antara Gandhara or both antara Gandhara and kakali Nishada.

For example, to create “Ni1_murcchana”, open the “Sa_murcchana” page and enter “nik” (i.e. N3) as the note to be placed on “sa”.
Raga scale types are stored in the “-cs.raga” Csound resource file. Images are available with a single click and scale structures are compared on the main page.
The entire process is summarized in the following table (Arnold 1982 p. 38):
| Step | Ma-grama chromatic murcchana starting from | Shuddha grama | Vikrit grama (antara) | Vikrit grama (antara + kakali) |
| 1 | Sa | kaphi1 | khamaj1 | bilaval1 |
| 2 | Ma1 | khamaj2 | bilaval2 | kalyan1 |
| 3 | Ni1 | bilaval3 | kalyan2 | marva1 |
| 4 | Ga1 | kalyan3 | marva2 | purvi1 |
| 5 | Dha1 | marva3 | purvi2 | todi1 |
| 6 | Re1 | purvi3 | todi2 | |
| 7 | Ma3 | todi3 | lalit1 bhairao1 | |
| 8 | Ni3 | lalit2 bhairao2 bhairavi1 | ||
| 9 | Ga3 | todi4 bhairavi2 | ||
| 10 | Dha3 | bhairavi3 | asavari1 | |
| 11 | Re3 | bhairavi4 | asavari2 | kaphi2 |
| 12 | Pa3 | asavari3 | kaphi3 | khamaj3 |
The use of this table deserves a graphical demonstration. For example, let us create a scale type “kalyan1″ based on the “Ma1_murcchana”. The table says that both “antara and kakali” should be selected. This means “antara Gandhara” which is “ga” in place of “gak” in the Ma-grama scale, and “kakali Nishada” which is “ni” in place of “nik” in the Ma-grama scale. This process is clear in the moving wheel model:
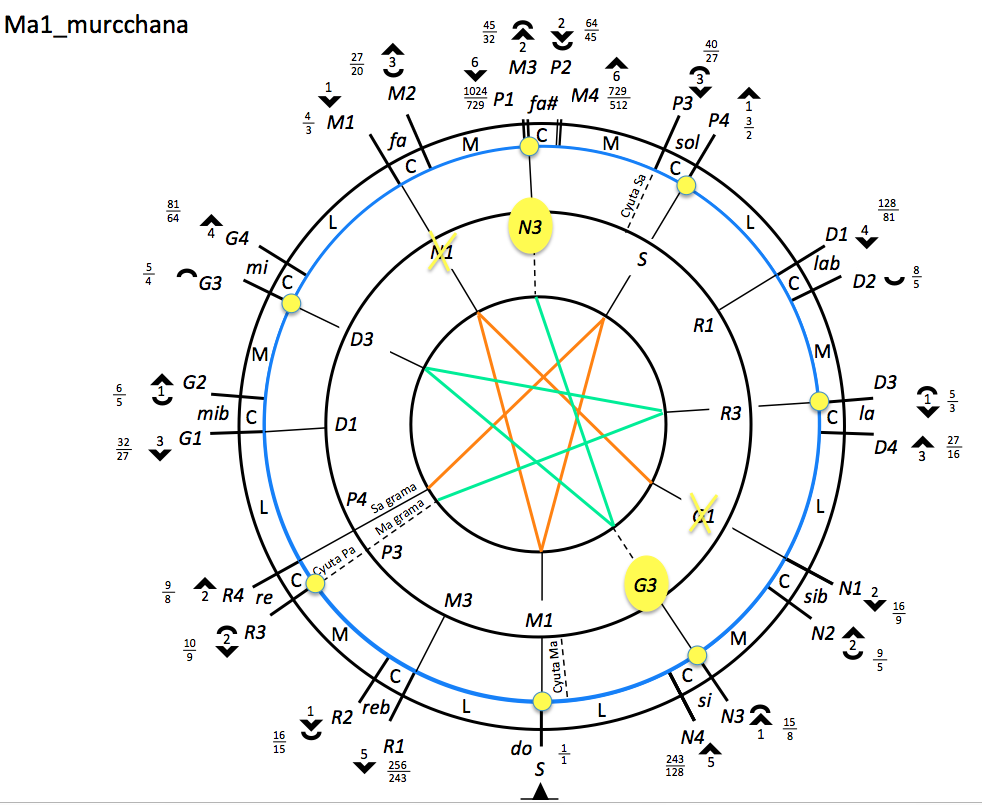
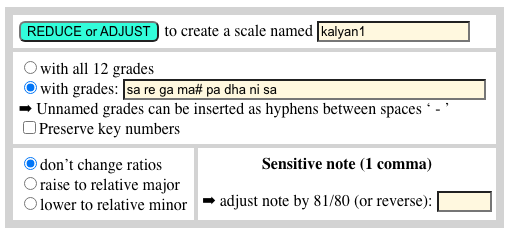
To make this selection and export the “kalyan1″ scale type, fill in the form on the “Ma1_murcchana” page as shown in the image.
Below is the resulting scale type.
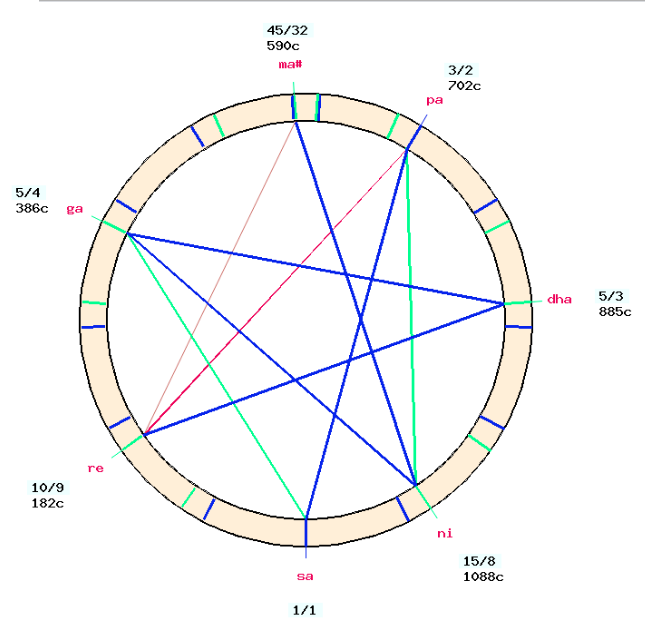
Remember that note positions expressed as whole-number frequency ratios are only a matter of convenience for readers familiar with western musicology. It would be more appropriate to follow the Indian convention of counting intervals in numbers of shruti-s. In this example, the interval between ‘sa’ and ‘ma’ is increased from 9 shruti-s (perfect fourth) to 11 shruti-s (tritone).
Arnold’s model is an extension of the murcchana system described in Natya Shastra because it accepts murcchana-s starting from notes that do not belong to the original (7-degree) Ma-grama, taken from its “chromatic version”: Dha1, Re1, Ma3, Ni3, Ga3. This extension is necessary to create scale types for Todi, Lalit and Bhairao that include augmented seconds.
In his 1982 paper (p. 39-41) Arnold linked his classification of scale types to the traditional list of jāti-s, the “ancestors of ragas” described in Sangita Ratnakara of Śārṅgadeva (Shringy & Sharma, 1978). Seven jāti-s are cited (p. 41), each of them being derived from a murcchana of the Ma-grama on one of its shuddha swara-s (basic notes).
Every jāti is associated with a note of relaxation (nyasa swara). In contemporary ragas, the nyasa swara is often found at the end of a phrase or a set of phrases. In Arnold’s interpretation, the same should define the murcchana from which the melodic type (jāti) is born. Since the names of the shuddha jatis are in fact tied to their nyasa swaras, this also suggests that they should be tied to the murcchanas belonging to those nyasa swaras (Arnold 1982 p. 40).
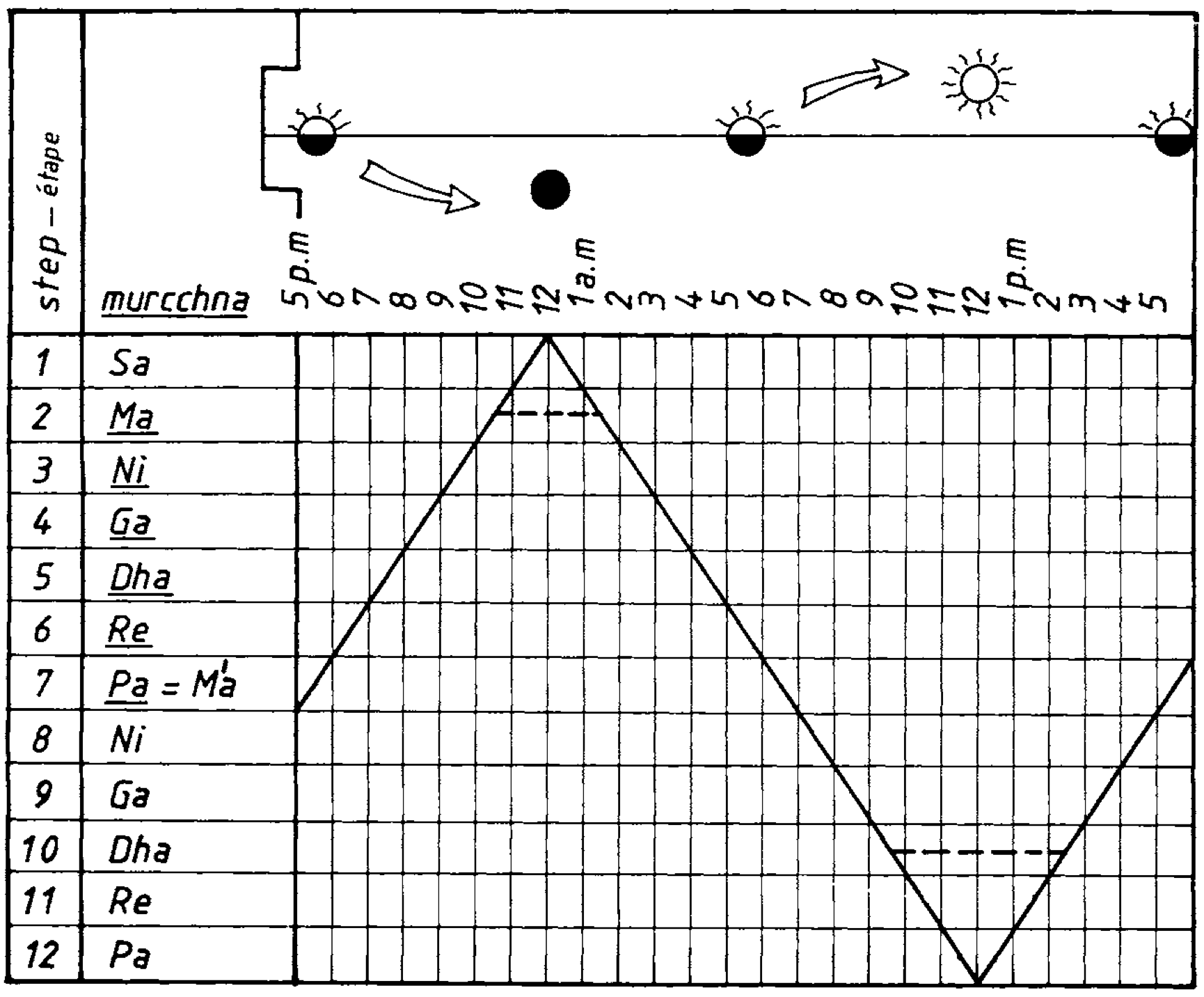
In other publications (notably Arnold & Bel 1985), Arnold used the cycle of 12 chromatic scales to suggest that the enharmonic positions of the notes could express states of tension or release linked to the changing ambience of the circadian cycle, thereby providing an explanation for the performance times assigned to traditional ragas. Low enharmonic positions would be associated with darkness and higher ones with daylight. Thus, ragas constructed using the Sa murcchana of the Ma-grama chromatic scale (all low positions, step 1) could be interpreted as being near midnight, while those that mix low and high positions (step 7) would carry the tensions of sunrise and sunset. Their sequence is a cycle because in the table above it is possible to jump from step 12 to step 1 by lowering all note positions by one shruti. This circularity is implied by the process called sadja-sadharana in musicological literature (Shringy & Sharma 1978).
A list of 85 ragas with performance times predicted by the model can be found in Arnold & Bel (1985). This hypothesis is indeed interesting — and it does hold for many well-known ragas — but we have never found the time to undertake a survey of musicians’ statements about performance times which might have assessed its validity.
Practice
Given scale types stored in the “-cs.raga” Csound resource file, Bol Processor + Csound can be used to check the validity of scales by playing melodies of ragas they are supposed to embody. It is also interesting to use these scales in musical genres unrelated with North Indian raga and “distort” them in every conceivable direction…
Choice of a raga

Public domain
We will take up the challenge of matching one of the four “todi” scales with two real performances of raga Todi.
Miyan ki todi is presently the most important raga of the Todi family and therefore often simply referred to as Todi […], or sometimes Shuddh Todi. Like Miyan ki malhar it is supposed to be a creation of Miyan Tansen (d. 1589). This is very unlikely, however, since the scale of Todi at the time of Tansen was that of modern Bhairavi (S R G M P D N), and the name Miyan ki todi first appears in 19th century literature on music.
Joep Bor (1999)
This choice is challenging for a number of reasons. Firstly, the four variants of “todi” scales are derived from a (questionable) extension of the grama-murcchana system. Then, the notes “ni” and “rek”, “ma#” and “dhak” are close to the tonic “sa” and the dominant “pa” and could be “attracted” by the tonic and dominant, thus disrupting the “geometry” of the theoretical scales in the presence of a drone.
Finally, and most importantly, the performer’s style and personal choices are expected to be at odds with this theoretical model. As suggested by Rao and van der Meer (2010, p. 693):
[…] it has been observed that musicians have their own views on intonation, which are handed down within the tradition. Most of them are not consciously aware of academic traditions and hence are not in a position to express their ideas in terms of theoretical formulations. However, their ideas are implicit in musical practice as musicians visualize tones, perhaps not as fixed points to be rendered accurately every time, but rather as tonal regions or pitch movements defined by the grammar of a specific raga and its melodic context. They also attach paramount importance to certain raga-specific notes within phrases to be intoned in a characteristic way.
We had already taken up the Todi challenge with an analysis of eight occurrences using the Melodic Movement Analyser (Bel 1988b). The analyser had produced streams of accurate pitch measurements which, after being filtered as selective tonagrams, were subjected to statistical analysis (Bel 1984; Bel & Bor 1984). The events included 6 performances of raga Todi and 2 experiments in tuning the Shruti Harmonium.
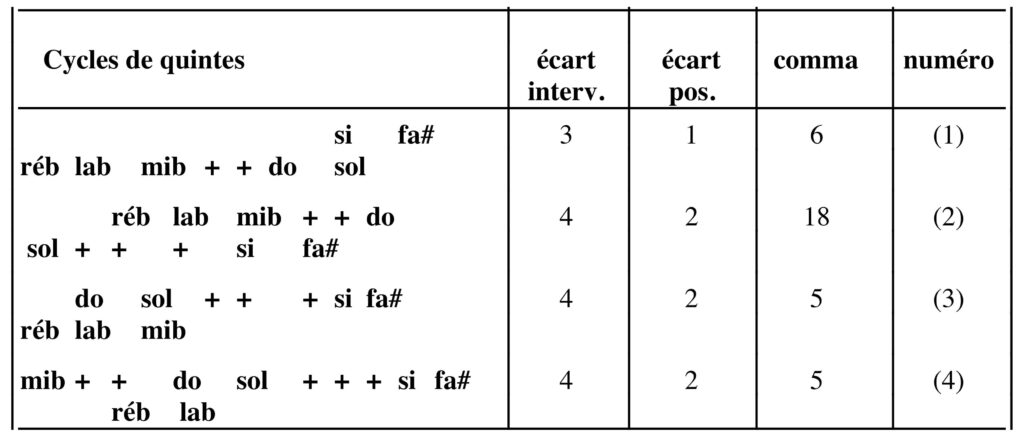
The second column is the standard deviation on intervals, and the third column is the standard deviation on positions relative to the tonic
The MMA analysis revealed a relatively high consistency of note positions, with standard deviations better than 6 cents for all notes except “ma#”, for which the deviation rose to 10 cents, still an excellent stability. Comparison of these results with the ‘flexible’ grama-murcchana model showed less than 4 cent standard deviation of intervals for 4 different scales in which the syntonic comma (pramāņa ṣruti) would be set at 6, 18, 5 and 5 cents respectively. In discussing tuning schemes, Wim van der Meer even suggested that musicians could “solve the problem” of a “ni-ma#” wolf fifth by tempering fifths over the “ni-ma#-rek-dhak” cycle (Bel 1988b p. 17).
Our conclusion was that no particular “tuning scheme” could be taken for granted on the basis of “raw” data. It would be more realistic to study a particular performance by a particular musician.
Choice of a musician

Credit সায়ন্তন ভট্টাচার্য্য - Own work, CC BY-SA 4.0
Working with the Shruti Harmonium naturally led us to meet Kishori Amonkar (1932-2017) in 1981. She was a leading exponent of Hindustani music, having developed a personal style that claimed to transcend classical schools (gharanas).
Most interestingly, she performed accompanied by a swara mandal (see picture), a zither that she tuned for each raga. Unfortunately we were not equipped to measure these tunings with sufficient accuracy. So we used the Shruti Harmonium to programme the intervals according to her instructions.
This experiment did not go well for two reasons. A technical one: on that day, a frequency divider (LSI circuit) on the harmonium was defective; until it was replaced, some programmed intervals were inaccessible. A musical one: the experiment showed that this precise harmonium was not suitable for tuning experiments with Indian musicians. The frequency ratios had to be entered on a small keyboard, a use too far removed from the practice of string tuning. This was a major incentive to design and build our “microscope for Indian music”, the Melodic Movement Analyser (MMA) (Bel & Bor 1984).
In the following years (1981-1984) MMA experiments took up all our time and revealed the variability (but not the randomness) of raga intonation. For this reason we could not return to tuning experiments. Today, a similar approach would be much easier with the help of the Bol Processor BP3… if only the expert musicians of that time were still alive!
Choice of a scale type
We need to decide between the four “todi” scale types produced by the murcchana-s of the Ma-grama chromatic scale. For this we can use the measurements of the Melodic Movement Analyser (Bel 1988b p. 15). Let us take average measurements and those of a performance by Kishori Amonkar. These are note positions (in cents) against the tonic “sa”.
| Note | Average | Standard deviation | Kishori Amonkar |
| rek | 95 | 4 | 96 |
| gak | 294 | 4 | 288 |
| ma# | 606 | 10 | 594 |
| pa | 702 | 1 | 702 |
| dhak | 792 | 3 | 792 |
| (dhak) | 806 | 3 | 810 |
| ni | 1107 | 6 | 1110 |
For the moment we will ignore “dhak” in the lower octave as it will be dealt with separately. Let us compare Kishori Amonkar’s results with the four scale types:
| Note | Kishori Amonkar | todi1 | todi2 | todi3 | todi4 |
| rek | 96 | 89 | 89 | 89 | 112 |
| gak | 288 | 294 | 294 | 294 | 294 |
| ma# | 594 | 590 | 590 | 610 | 610 |
| pa | 702 | 702 | 702 | 700 | 702 |
| dhak | 792 | 792 | 792 | 792 | 814 |
| ni | 1110 | 1088 | 1109 | 1109 | 1109 |
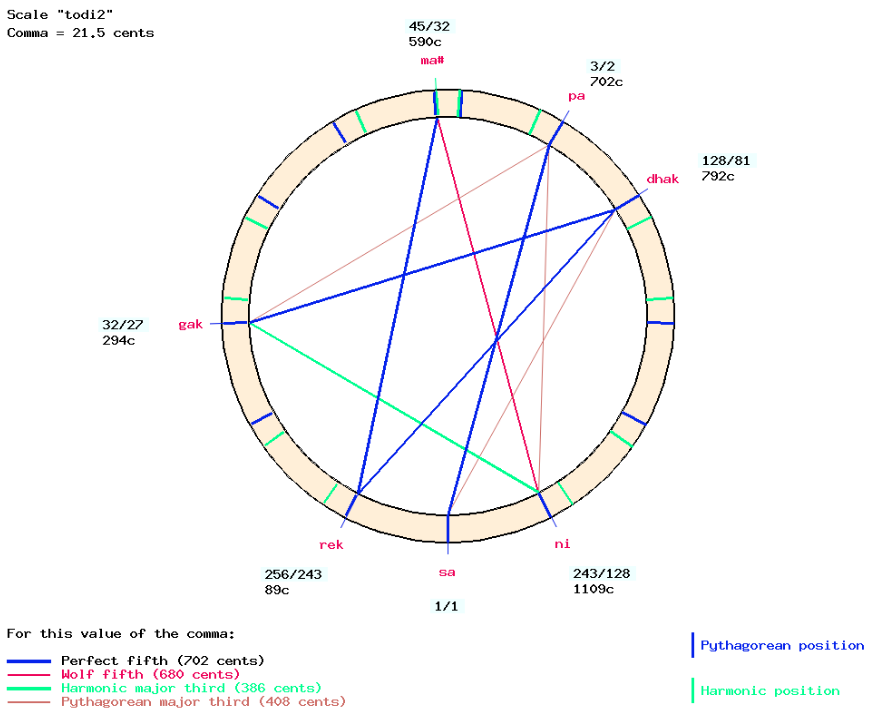
There are several ways of finding the best match for musical scales: either by comparing scale intervals or by comparing note positions in relation to the base note (tonic). Because of the importance of the drone, we will use the second method. The choice is simple here. Version “todi1″ can be discarded because of “ni”, the same with “todi3″ and “todi4″ because of “ma#”. We are left with “todi2″ which has a very good match, even with the measurements of performances by other musicians.
Adjustment of the scale
The largest deviations are on “rek” which was sung 7 cents higher than the predicted value and “gak” which was sung 6 cents lower. Even a 10-cent deviation is practically impossible to measure on a single note sung by a human, including a high-profile singer like Kishori Amonkar; the best resolution used in speech prosody is greater than 12 cents.
Any “measurement” of the MMA is an average of values along the rare stable melodic steps. It may not be representative of the “real” note due to its dependence on note treatment: if the note’s approach is in a range on the lower/higher side, the average will be lower/higher than the target pitch.
Therefore, it would be acceptable to declare that the scale type “todi2″ corresponds to the performance. However, let us show how the model can be modified to reflect the measurements more accurately.

First we duplicate “todi2″ to create “todi-ka” (see picture). Note positions are identical in both versions.
Looking at the picture of the scale (or the numbers on its table), we can see that all the note positions except “ma#” are Pythagorean. The series to which a note belongs is indicated by the colour of its pointer: blue for Pythagorean and green for harmonic.
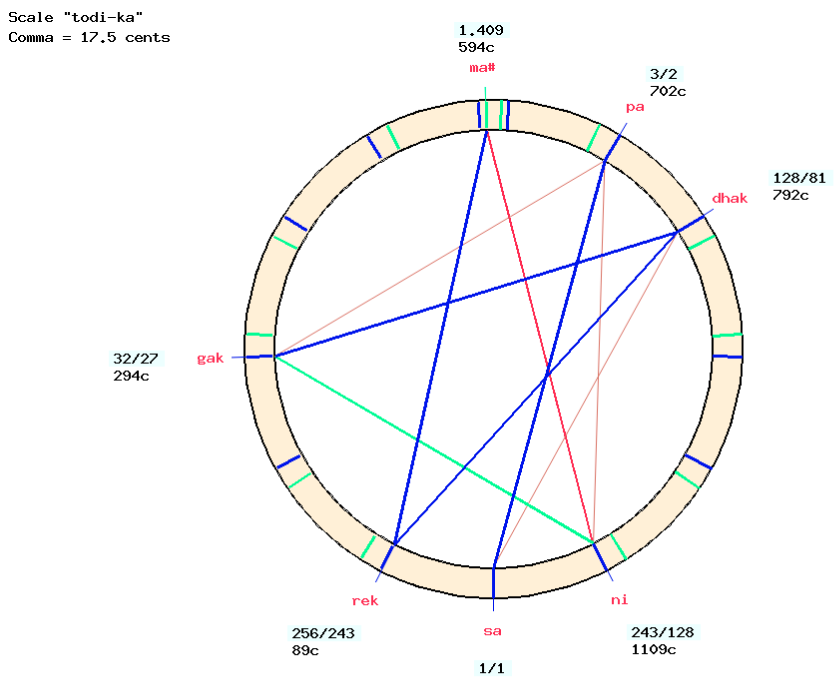
This means that changing the size of the syntonic comma — in strict accordance with the grama-murcchana model — will only adjust “ma#”. To change the position of “ma#” from 590 to 594 cents (admittedly a ridiculous adjustment) we need to reduce the size of the syntonic comma by the same amount. This can be done at the bottom right of the “todi-ka” page, changing the syntonic comma to 17.5 cents, a change confirmed by the new image.
A table on the “todi-ka” page shows that the “rek-ma#” interval is still considered a “perfect” fifth, even though it is smaller by 6 cents.
It may not be obvious whether the syntonic comma needs to be increased or decreased to fix the position of “ma#”, but it is easy to try the other way in case the direction was wrong.
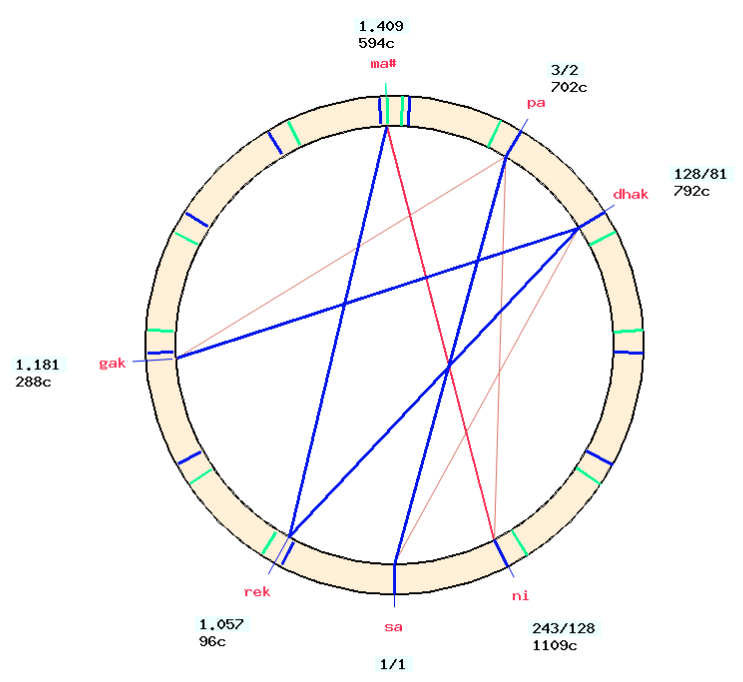
Other adjustments deviate from the “pure” model. These result in changing frequency ratios in the table on the “todi-ka” page. An increase in “rek” from 89 to 96 cents requires an increase of 7 cents, corresponding to a ratio of 2(7/1200) = 1.00405. This changes the position of “rek” from 1.053 to 1.057.
In the same way, a reduction in “gak” from 294 to 288 cents requires a reduction of 6 cents, giving a ratio of 2(-6/1200) = 0.9965. This brings the position of “gak” from 1.185 to 1.181.
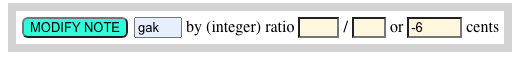
Fortunately, these calculations are done by the machine: use the “MODIFY NOTE” button on the scale page.
The picture shows that the information of “rek” and “gak” belonging to Pythagorean series (blue line) is preserved. The reason for this is that whenever a frequency ratio is modified by its floating-point value, the machine checks whether the new value is close to an integer ratio of the same series. For example, changing “rek” back to 1.053 would restore its ratio to 256/243. Accuracy better than 1‰ is required for this adjustment.
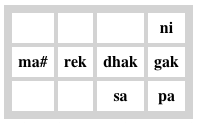
A tuning scheme for this scale type is suggested by the machine. The graphical representation shows that “ni” is not consonant with “ma#” as their interval is 684 cents, close to a wolf fifth of 680 cents. Other notes are arranged on two cycles of perfect fifths. Interestingly, raising “rek” by 7 cents brought the “rek-ma#” fifth back to its perfect size (702 cents).
Again, these are meaningless adjustments for a vocal performance. We are just showing what to do when necessary.
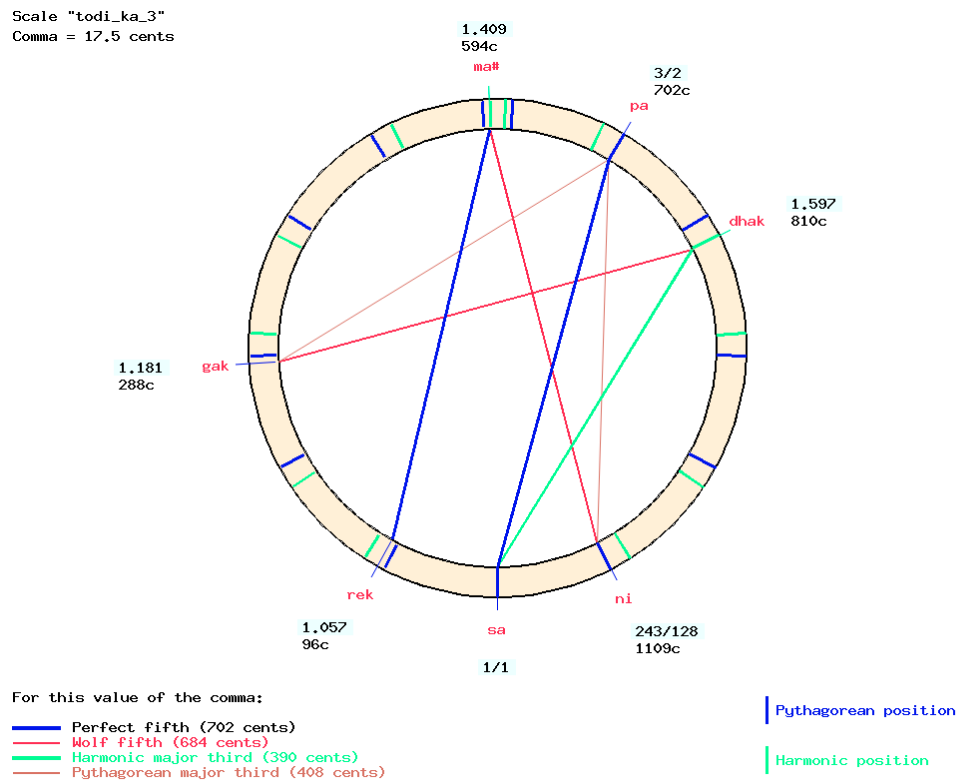
The remaining adjustment will be that of the “dhak” in the lower octave. To do this, we will duplicate the previous scale, renaming it “todi_ka_4″ to indicate that it is designed for the 4th octave. In the new scale, called “todi_ka_3″, we raise “dhak3” by 810 -792 = 18 cents.
This raises its position from 1.58 to 1.597. Note that this puts it exactly in a position in the harmonic series since the syntonic comma is 17.5 cents.
In addition, “dhak-sa” is now a harmonic major third — with a size of 390 cents to match the 17.5 cents comma. This is certainly significant in the melodic context of this raga, which is one reason why all the musicians made the same size adjustment in their tuning experiments.
This case is a simple illustration of raga intonation as a trade-off between harmonicity with respect to the drone and the need for consonant melodic intervals. It also shows that the Shruti Harmonium could not follow the practice of the musicians because its scale ratios were replicated in all octaves.
Choice of a recording
We don’t have the recording on which the MMA analysis was made. One problem with old tape recordings is the unreliability of the speed of tape transport. Also, on a long recording, the frequency of the tonic can change slightly due to variations in room temperature, which affects the instruments — including the dilation of the tape…
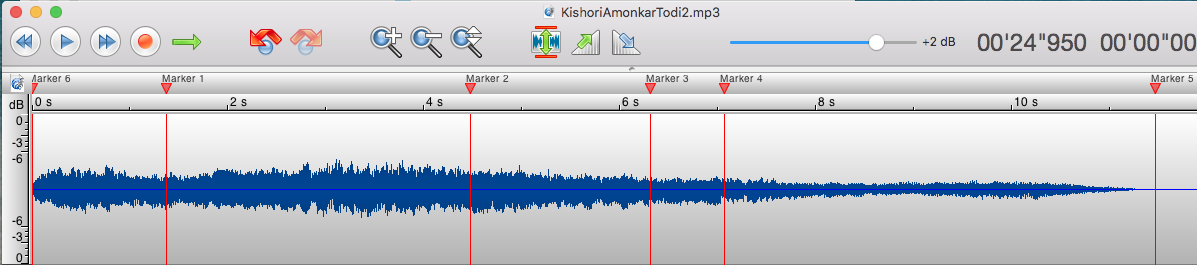
In order to try to compare scales a with real performances, and to examine extremely small “deviations” (which have little musical significance, in any case), it is therefore safer to work with digital recordings. This was the case with Kishori Amonkar’s Todi, recorded in London in early 2000 for the Passage to India collection, and available free of copyright (link on Youtube). The following is based on this recording.
Setting up the diapason
Let us create the following “-gr.tryRagas” grammar:
-se.tryRagas
-cs.raga
S --> _scale(todi_ka_4,0) sa4
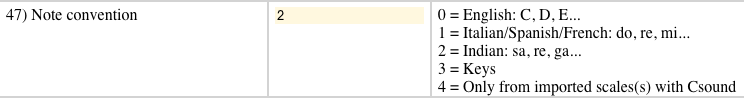
In “-se.tryRagas” the note convention should be set to “Indian” so that “sa4” etc. is accepted even when no scale is specified.
The grammar calls “-cs.raga”, which contains the definitions of all the scale types created by the procedure described above. Unsurprisingly, it does not play the note “sa” at the frequency of the recording. We therefore need to measure the tonic in order to adjust the frequency of “A4” (diapason) in “-se.tryRagas” accordingly. There are several ways to do this with increasing accuracy.
A semitone approximation can be obtained by comparing the recording with notes played on a piano or any electronic instrument tuned with A4 = 440 Hz. Once we have found the key that is closest to “sa” we calculate its frequency ratio to A4. If the key is F#4, which is 3 semitones lower than A4, the ratio is r = 2(-3/12) = 0.840. To get this frequency on “sa4” we would therefore have to adjust the frequency of the diapason (in “-se.tryRagas”) to:
440 * r * 2(9/12) = 440 * 2((9-3)/12) = 311 Hz
A much better approximation is obtained by extracting a short occurrence of “sa4” at the very beginning of the performance:
Then select a seemingly stable segment and extend the time scale to get a visible signal:
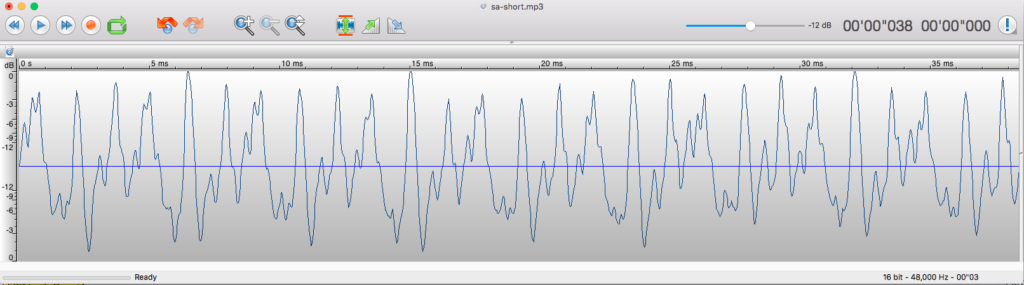
This sample contains 9 cycles for a duration of 38.5 ms. The fundamental frequency is therefore 9 * 1000 / 38.5 = 233.7 Hz. Consequently, adjust the diapason in “-se.tryRagas” to 233.7 * 2(9/12) = 393 Hz.
The last step is a fine tuning by comparing the production of the notes in the grammar by ear with the recording of “sa4” played in a loop. To do this, we produce the following sequence:
S --> _pitchrange(500) _tempo(0.2) Scale _pitchbend(-15) sa4 _pitchbend(-10) sa4 _pitchbend(-5) sa4 _pitchbend(-0) sa4 _pitchbend(+5) sa4 _pitchbend(+10) sa4 _pitchbend(+15) sa4 _pitchbend(+20) sa4
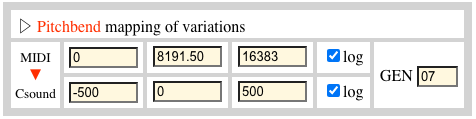
These are eight occurrences of “sa4” played at slightly increasing pitches adjusted by the pitchbend. First make sure that the pitchbend is measured in cents: this is specified in the instrument “Vina” called by “-cs.raga” and the Csound orchestra file “new-vina.orc”.
Listening to the sequence may not reveal any pitch differences, but these will be apparent to a trained ear when superimposed on the recording:
➡ This is a stereo recording. Use headphones to hear the song and the sequence of plucked notes on separate channels
One of the four occurrences sounds best in tune. Let us assume that the best match is on _pitchbend(+10). This means that the diapason should be raised by 10 cents. Its new frequency would therefore be 393 * 2(10/1200) = 395.27 Hz.
In fact the best frequency is 393.22 Hz, which means that the second evaluation (which gave 393 Hz) was fair — and the singers’ voices very reliable! Now we can check the frequency of “sa4” on the Csound score:
; Csound score
f1 0 256 10 1 ; This table may be changed
t 0.000 60.000
i1 0.000 5.000 233.814 90.000 90.000 0.000 -15.000 -15.000 0.000 ; sa4
i1 5.000 5.000 233.814 90.000 90.000 0.000 -10.000 -10.000 0.000 ; sa4
i1 10.000 5.000 233.814 90.000 90.000 0.000 -5.000 -5.000 0.000 ; sa4
i1 15.000 5.000 233.814 90.000 90.000 0.000 0.000 0.000 0.000 ; sa4
i1 20.000 5.000 233.814 90.000 90.000 0.000 5.000 5.000 0.000 ; sa4
i1 25.000 5.000 233.814 90.000 90.000 0.000 10.000 10.000 0.000 ; sa4
i1 30.000 5.000 233.814 90.000 90.000 0.000 15.000 15.000 0.000 ; sa4
i1 35.000 5.000 233.814 90.000 90.000 0.000 20.000 20.000 0.000 ; sa4
s
These methods could actually be summarised by the third: use the grammar to produce a sequence of notes in a wide range to determine an approximate pitch of “sa4” until the small range for the pitchbend (± 200 cents) is reached. Then play sequences with pitchbend values in increasing accuracy until no discrimination is possible.
In a real exercise it would be safe to check the measurement of “sa4” against occurrences in several parts of the recording.
This approach is too demanding in terms of accuracy for the analysis of a vocal performance, but it will be noticeable when working with a long-stringed instrument such as the rudra veena. We will demonstrate this with Asad Ali Kan’s performance.
Matching phrases of the performance
We are now ready to check whether note sequences produced by the model would match similar sequences in the recording.
First we try a sequence with the emphasis on “rek”. The following sequence of notes is produced by the grammar:
S --> KishoriAmonkar1
KishoriAmonkar1 --> Scale _ {2, dhak3 sa4 ni3 sa4} {7, rek4} _ {2, dhak3 sa4 ni3 dhak3} {2, dhak3 _ ni3 sa4} {5, rek4}
Scale --> _scale(todi_ka_3,0)
Below is the phrase sung by the musicians (position 0′50″) then repeated in superposed form with the sequence produced by the grammar:
➡ This is a stereo recording. Use headphones to hear the song and sequence of plucked notes on separate channels
In this example, the scale “todi_ka_3″ has been used because of the occurrence of short instances of “dhak3”. The position of “rek” is identical in the 3d and 4th octaves. The blending of the voice with the plucked instrument is notable in the last held note.
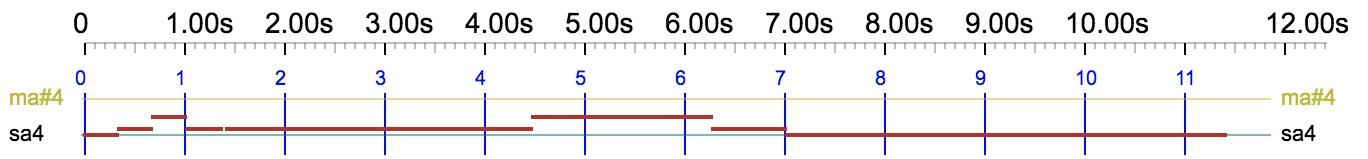
In the next sequence (location 1′36″) the position of “gak4” is estimated. The grammar is as follows:
S --> KishoriAmonkar2
KishoriAmonkar2 --> Scale {137/100, sa4 rek4 gak4 rek4} {31/10, rek4} {18/10, gak4} {75/100,rek4} {44/10, sa4}
Scale --> _scale(todi_ka_4,0)
This time, the scale “todi_ka_4″ was chosen, even though it had no effect on the intonation since “dhak” is missing.
A word about building the grammar: we looked at the signal of the recorded phrase and measured the (approximate) duration of the notes: 1.37s, 3.1s, 1.8s, 7.5s, 4.4s. We then converted these durations into integer ratios — fractions of the basic tempo whose period is exactly 1 second, as specified in “-se.tryRagas”: 137/100, 31/10 etc.
Below is a pianoroll of the sequence produced by the grammar:
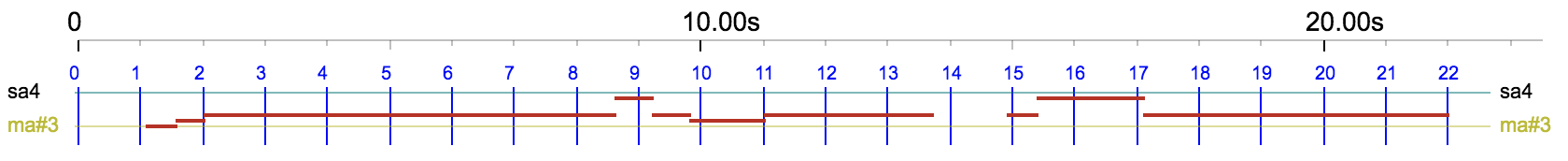
No we try a phrase with a long pause on “dhak3” (location 3′34″), which proves that the scale “todi_ka_3″ perfectly matches this occurrence of “dhak”:
S --> KishoriAmonkar3
KishoriAmonkar3 --> scale(todi_ka_3,0) 11/10 {19/20, ma#3 pa3} {66/10,dhak3} {24/10, ni3 dhak3 pa3 }{27/10,dhak3} 12/10 {48/100,dhak3}{17/10,ni3}{49/10,dhak3}
Early occurrence of “ma#4” (location 11′38″):
S --> KishoriAmonkar4
KishoriAmonkar4 --> _scale(todi_ka_4,0) 4/10 {17/10, ni3}{26/100,sa4}{75/100,rek4}{22/100,gak4}{17/10,ma#4}{16/100,gak4}{34/100,rek4}{56/100,sa4}{12/100,rek4}{84/100,gak4}{27/100,rek4}{12/10,sa4}
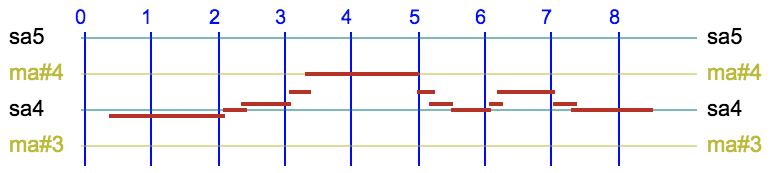
Reaching “dhak4” (location 19′46″):
S --> KishoriAmonkar5
KishoriAmonkar5 --> _scale(todi_ka_4,0) 13/10 {16/10,ma#4}{13/10,gak4}{41/100,ma#4}{72/100,ma#4 dhak4 ma#4 gak4 ma#4}{18/10,dhak4}{63/100,sa4}{90/100,rek4}{30/100,gak4}{60/100,rek4}{25/100,sa4}{3/2,rek4}
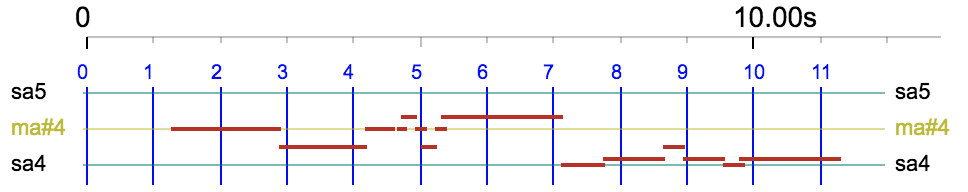
With a light touch of “pa4” (location 23′11″):
S --> KishoriAmonkar6
KishoriAmonkar6 --> _scale(todi_ka_4,0) 28/100 {29/100,ma#4}{40/100,dhak4}{63/100,ni4 sa5 ni4}{122/100,dhak4}{64/100,pa4}{83/100,ma#4}{44/100,pa4}{79/100,dhak4}
Pitch accuracy is no surprise in Kishori Amonkar’s performances. With a keen awareness of “shruti-s”, she would sit on the stage and pluck her swara mandal, carefully tuned for each raga.
A test with the rudra veena
Asad Ali Khan (1937-2011) was one of the last performers of the rudra veena at the end of the 20th century and a very supportive participant in our scientific research on raga intonation.
➡ An outstanding presentation of Asad Ali Khan and his idea of music is available in a film by Renuka George.
Pitch accuracy on this instrument is such that we have been able to identify tiny variations that are controlled and significant in the context of the raga. See for example Playing with Intonation (Arnold 1985). To measure vibrations below the audible range, we occasionally placed a magnetic pickup near the last string.
Below are the statistics of the Melodic Movement Analyser measurements of the raga Miyan ki Todi interpreted by Asad Ali Khan in 1981. The second column contains measurements of his tuning of the Shruti Harmonium during an experiment. The columns on the right show the predicted note positions according to the grama-murchana model with a syntonic comma of ratio 81/80. As shown in Kishori Amonkar’s performance above, “dhak” can take different values depending on the octave.
| Note | Asad Ali Khan performing | Asad Ali Khan tuning | todi1 | todi2 | todi3 | todi4 |
| rek | 99 | 100 | 89 | 89 | 89 | 112 |
| gak | 290 | 294 | 294 | 294 | 294 | 294 |
| ma# | 593 | 606 | 590 | 590 | 610 | 610 |
| pa | 702 | 702 | 702 | 702 | 700 | 702 |
| dhak3 | 795 | 794 | 792 | 792 | 792 | 814 |
| dhak2 | 802 | |||||
| ni | 1105 | 1108 | 1088 | 1109 | 1109 | 1109 |
Again, the best match would be the “todi2″ scale with a syntonic comma of 17.5 cents. We created two scales, “todi_aak_2″ and “todi_aak_3″ for the 2nd and 3rd octaves.
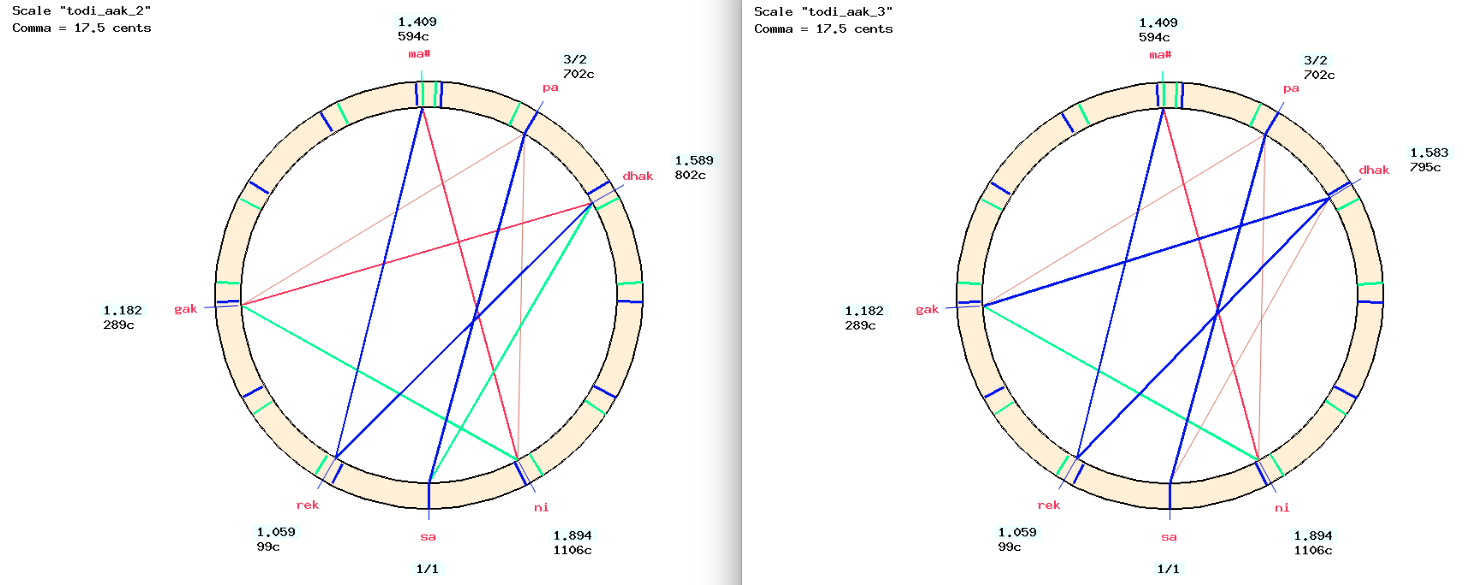
The scale constructed during the Shruti Harmonium experiment is less relevant because of the influence of the experimenter playing the scale intervals with a low-attracting drone (produced by the machine). In his attempt to resolve the dissonance in the scale — which always contained a wolf fifth and several Pythagorean major thirds — Khan saheb ended up with a tuning identical to the initial one, but one comma lower. This was not a musically significant situation!
The scale “todi_aak_2″ (in the low octave) contains interesting intervals (harmonic major thirds) which allows us to anticipate effective melodic movements. The tuning scheme summarises these relationships.
We now take fragments of Asad Ali Khan’s performance of Todi (2005) available on Youtube (follow this link).
The performance begins in the lower octave, so with the scale “todi_aak_2″. The frequency of Sa was measured at 564.5 Hz using the method described above.
Let us start with a simple melodic phrase repeated twice, the second time superimposed on the note sequence produced by the grammar.
➡ This is a stereo recording. Use headphones to hear the song and the sequence of plucked notes on separate channels
S --> AsadAliKhan1
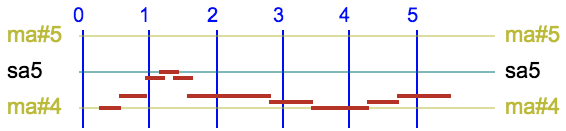
AsadAliKhan1 --> _scale(todi_aak_2,0) 45/100 {69/10,sa3} {256/100,dhak2} {78/10,dhak2} {12/10,sa3 ni2 rek3&} {48/10,&rek3} {98/100,sa3 ni2 sa3&} {27/10,&sa3}
This grammar contains an unusual character ‘&’, which is used to concatenate sound objects (or notes) across the boundaries of polymetric expressions (between curly brackets). This makes it possible to play the final “rek3” and “sa3” as continuous notes. This continuity can be seen in the graph below:
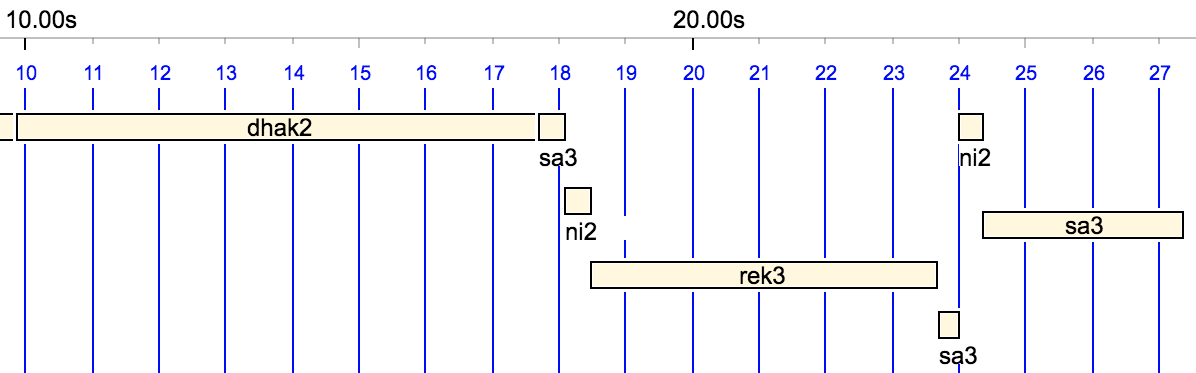
It is time to make sure that fine tuning and adjusting scales are more than just an intellectual exercise… After all, the main difference between scales “todi_aak_2″ and “todi_aak_3″ is that “dhak” is 7 cents higher in “todi_aak_2″, which means a third of a comma! To check the effect of the fine-tuning, listen to the overlay twice, once with “todi_aak_3″ and the second time with “todi_aak_2″:
To check the difference between these two versions of “dhak2”, we can play them one after the other and then superimpose them:
S --> _tempo(1/2) _scale(todi_aak_3,0) dhak2 _scale(todi_aak_2,0) dhak2 {_scale(todi_aak_3,0) dhak2, _scale(todi_aak_2,0) dhak2}
With fundamental frequencies of 132.837 Hz and 133.341 Hz, the beat frequency (of the sine waves) would be 133.341 - 132.837 = 0.5 Hz. The perceived beat frequency is higher because of the interference between the higher partials. This suggests that a difference of 7 cents is not irrelevant in the context of notes played by a long-stringed instrument (Arnold 1985).
More in the lower octave:
S --> AsadAliKhan2
AsadAliKhan2 --> scale(todi_aak_2,0) _volume(64) _pitchrange(500) _pitchcont 93/100 {81/10,pa2}{38/10,pa2 gak2 pa2 dhak2 pa2 }{19/10,gak2}{43/10, _pitchbend(0) rek2 _pitchbend(-100) rek2&} _volumecont _volume(64) {2, _pitchbend(-100) &rek2} _volume(0) _volume(64) {23/10,ni2__ dhak2}{103/100,sa3&}{4,&sa3} 15/10 _volume(64) {38/10,sa3} _volume(0)
As “sa2” is outside the range of the Csound instrument “Vina”, it is played here as “rek2” with a pitchbend correction of one semitone.
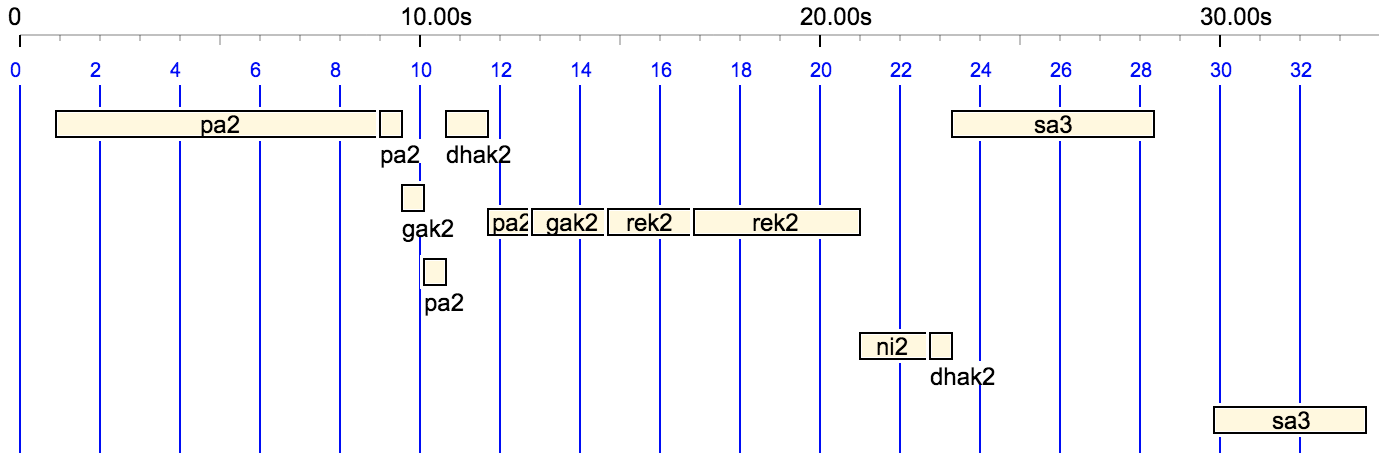
The rendering of phrases in the lower octave is very approximate because of the predominance of meend (pulling the string). Some effects can be better imitated using performance controls — see Sarasvati Vina, for example — but this requires a mastery of the real instrument in order to design patterns of musical “gestures” rather than sequences of sound events… Imitating the melodic intricacy of a raga is not the subject of this page; we are merely checking the relevance of scale models to the “tonal skeleton” of ragas.
Accidental notes
Raga scales extracted from murchana-s of the Ma-grama chromatic scale (see above) contain only notes that are presumed to belong to the raga. They cannot accommodate accidental notes or the scales used in the common practice of mixing ragas.
Let us take, for example, a fragment of the previous example, which was poorly represented by the sequence of notes produced by the grammar. (We learn from our mistakes!) We might be tempted to replace the expression {38/10, pa2 gak2 pa2 dhak2 _ pa2 _} with {38/10, pa2 ga2 pa2 dhak2 _ pa2 _}, using “ga2” which does not belong to the scale “todi_aak_2″. Unfortunately, this results in an error message:
ERROR Pitch class ‘4’ does not exist in _scale(todi_aak_2). No Csound score produced.
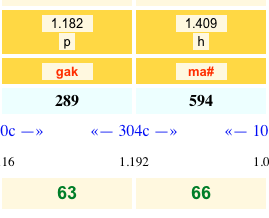
This amounts to saying that scale “todi2″ contains no mapping of key #64 to “ga” — nor key # 65 to “ma”, see figure.
To solve this problem we can recall that the scale “todi2″ was extracted from “Re1_murcchana”. The latter contains all the notes of a chromatic scale in addition to those extracted. Therefore it is sufficient to replace “_scale(todi_aak_2,0)” with “_scale(Re1_murcchana,0)” in this section:
_scale(Re1_murcchana,0) {38/10, pa2 ga2 pa2 dhak2 _ pa2 _} _scale(todi_aak_2,0) etc.
The scale editor takes care of assigning a key number to each note based on the chromatic scale if a standard English, Italian/French or Indian note convention is used. In other cases this mapping should be done by hand. Designers of microtonal scales should be aware of key mappings when using custom names for “notes”.
Another problem is that in “todi_aak_2″ note “dhak” has been raised from 792 to 810 cents, which is not its value in “Re1_murcchana”. This can be fixed by creating another variant of the scale with this correction, or simply using the pitchbend to modify “dhak2” — in which case the same pitchbend could have been used to raise “gak2” in the first place.
Finally, the best way to avoid this problem would be to use the source chromatic scale “Re1_murcchana”, a murcchana of Ma-grama, to construct raga scales even though some notes will never be used.
To conclude…
This whole discussion has been technical. There is no musical relevance in trying to associate plucked notes with very subtly ornamented melodic movements. The last excerpt (2 repetitions) will prove — if it is needed at all — that the intonation of Indian ragas is much more than a sequence of notes in a scale, whatever its accuracy:
S --> AsadAliKhan3
AsadAliKhan3 --> scale(todi_aak_3,0) 94/100 {26/10,sa3}{23/10,sa3 rek3 gak3}{195/100,ma#3}{111/100,rek3}{24/10,rek3 sa3}{33/10,sa3 sa3}{71/100,rek3}{76/100,gak3}{71/100,dhak3 ma#3}{176/100,dhak3}{75/100,sa4}{27/10,dhak3__ sa4}{620/100,sa4 dhak3 ma#3 dhak3 ma#3 gak3 _ ma#3 dhak3 dhak3&}{266/100,&dhak3}{672/100,pa3____ pa3_ pa3 pa3 pa3__}{210/100,pa3 ma#3 pa3 dhak3}{222/100,dhak3}{163/100,gak3 ma#3}{426/100,gak3_ rek3____}{346/100,sa3}
➡ This is a stereo recording. Use headphones to hear the song and the sequence of plucked notes on separate channels
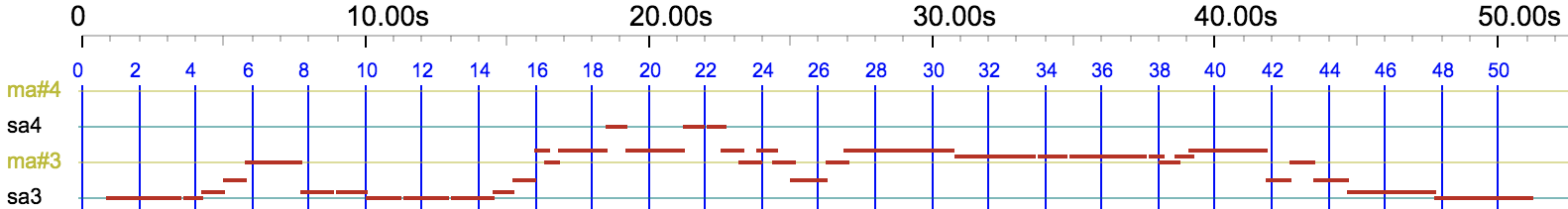
Listen to Asad Ali Khan’s actual performance of raga Todi to appreciate its expressive power!
Attempting to follow the intricacies of alankara (note treatment) with a simplistic notation of melodic phrases demonstrates the disconnection between ‘model-based’ experimental musicology and the reality of musical practice. This explains why we have relied on descriptive models (e.g. automatic notation) captured by the Melodic Movement Analyser or computer tools such as Praat, rather than attempting to reconstruct melodic phrases from theoretical models. Experiments with scales deal with the “skeletal” nature of intonation, which is a necessary but not sufficient parameter for describing melodic types.
All examples shown on this page are available in the bp3-ctests-main.zip sample set shared on GitHub. Follow the instructions on Bol Processor ‘BP3’ and its PHP interface to install BP3 and learn its basic operation. Download and install Csound from its distribution page.
Bernard Bel — Dec. 2020
References
Arnold, E.J.; Bel, B. L’intonation juste dans la théorie ancienne de l’Inde : ses applications aux musiques modale et harmonique. Revue de musicologie, JSTOR, 1985, 71e (1-2), p.11-38.
Arnold, E.J. A Mathematical model of the Shruti-Swara-Grama-Murcchana-Jati System. Journal of the Sangit Natak Akademi, New Delhi 1982.
Arnold, E.J.; Bel, B. A Scientific Study of North Indian Music. NCPA Quarterly Journal, vol. XII Nos. 2 & 3, Bombay 1983.
Arnold, W.J. Playing with Intonation. ISTAR Newsletter Nr. 3-4, June 1985 p. 60-62.
Bel, B. Musical Acoustics: Beyond Levy’s “Intonation of Indian Music”. ISTAR Newsletter Nr 2, April 1984.
Bel, B. A Mathematical Discussion of the Ancient Theory of Scales according to Natyashastra. Note interne, Groupe Représentation et Traitement des Connaissances (CNRS), March 1988a.
Bel, B. Raga : approches conceptuelles et expérimentales. Actes du colloque “Structures Musicales et Assistance Informatique”, Marseille 1988b.
Bel, B.; Bor, J. Intonation of North Indian Classical Music: working with the MMA. National Center for the Performing Arts. Video on Dailymotion, Mumbai 1984.
Bharata. Natya Shastra. There is no currently available English translation of the first six chapters of Bharata’s Natya Shastra. However, most of the information required for this interpretation has been reproduced and commented by Śārṅgadeva in his Sangita Ratnakara (13th century AD).
Bor, J.; Rao, S.; van der Meer, W.; Harvey, J. The Raga Guide. Nimbus Records & Rotterdam Conservatory of Music, 1999. (Book and CDs)
Bose, N.D. Melodic Types of Hindustan. Bombay, 1960: Jaico.
Hirst, D. Speech Prosody - Chapter 8. Modelling Speech Melody. Preprint, 2022.
Rao, S.; Van der Meer, W. The Construction, Reconstruction, and Deconstruction of Shruti. Hindustani music: thirteenth to twentieth centuries (J. Bor). New Delhi, 2010: Manohar.
Shringy, R.K.; Sharma, P.L. Sangita Ratnakara of Sarngadeva: text and translation, vol. 1, 5: 7-9. Banaras, 1978: Motilal Banarsidass. Source in the Web Archive.
Van der Meer, W.; Rao, S. Microtonality in Indian Music: Myth or Reality. Gwalior, 2009: FRSM.
Van der Meer, W. Gandhara in Darbari Kanada, The Mother of All Shrutis. Pre-print, 2019.
Van der Meer, W.; Rao, S. MUSIC IN MOTION. The Automated Transcription for Indian Music (AUTRIM) Project by NCPA and UvA, 2010.
Van der Meer, W. The AUTRIM Project, Music in Motion, 2020.
Initial feedback
This project began in 1980 with the founding of the International Society for Traditional Arts Research (ISTAR) in New Delhi, India. We had produced joint articles and proposals which enabled us (Arnold and Bel) to receive a grant from the International Fund for the Promotion of Culture (UNESCO). A booklet of ISTAR projects was then printed in Delhi, and a larger team received support from the Sangeet Research Academy (SRA, Calcutta/Kolkata), the Ford Foundation (USA) and the National Centre for the Performing Arts (NCPA, Bombay/Mumbai).
The following are extracts from letters of support received during this initial period — after the construction of the Shruti Harmonium and during the design of the Melodic Movement Analyser. (ISTAR booklet, 1981 pages 20-22)
In fact, the full potential of this approach can only be realised now, taking advantage of the (virtually unlimited) digital devices that are replacing the hardware we created for this purpose 40 years ago!
The work of Mr. Arnold and Mr. Bel, as much from the theoretical point of view as from the point of view of the practical realization, appears to be one of the best of these last years, as concerns the musical analysis of the classical music of India…
— Iégor REZNIKOFF, Director, UER of Philosophy, History of Art and Archeology, Mathematics, University of Paris X - Nanterre.
I consider that this work presents the greatest interest and is capable of considerably advancing the understanding of the problem of the use of micro-intervals in the music of India, and more generally, that of the intervals found in different modal musics.
— Gilbert ROUGET, Director of Research at CNRS, in charge of the Department of Ethnomusicology at the Musée de l’Homme, Paris.
The ideas and conceptions of Mr. Arnold and Mr. Bel seem tome to have the utmost interest musically because they rest not just on pure theories; but on a profound understanding of melodic and modal music, etc. The project which Mr. Bel presented to me could bring about a realization much more interesting and effective than that of the various “melographs” which have been proposed…
— Émile LEIPP, Director of Research at the CNRS, Director of Laboratoire d’Acoustique, University of Paris VI.
The project entitled “A Scientific study of the modal music of North India” undertaken by E. James Arnold and Bernard Bel is very interesting and full of rich potentials. This collaboration of mathematics and physical sciences as well as engineering sciences on the one hand, and Indology and Indian languages, musicology, as well as applied music on the other hand can be reasonably expected to yield fascinating results.
— Dr. Prem Lata SHARMA, Head of the Department of Musicology and Dean of the Faculty of Performing Arts, Banaras Hindu University.
Mr. Arnold’s work on the logic of the grama-murcchana system and its ‘applications’ to current Indian music is a most stimulating and original piece of investigation. Mr. Arnold’s research and he and his partner (Mr. Bel)‘s work have immense implications for music theory and great value for theoretical study of Indian music.
— Bonnie C. WADE, Associate Professor of Music, University of California
Looking forward into the future, it (the Shruti harmonium) opens up a new field to composers who wish to escape from the traditional framework in which they are trapped, by virtue of the multiplicity of its possibilities for various scales, giving hence a new material.
— Ginette KELLER, Grand Prize of Rome, Professor of Musical Analysis and Musical Aesthetics, ENMP and CNSM, Paris.
I was astonished to listen to the “shrutis” (microtones) produced by this harmonium which they played according to my suggestion, and I found the ‘gandhars’, ‘dhaivats’, ‘rikhabs’ and ‘nikhads’ (3rds, 6ths, 2nds and 7ths) of ragas Darbari Kanada, Todi, Ramkali and Shankara to be very correctly produced exactly as they could be produced on my violin.
— Prof. V.G. JOG, Violinist, recipient of the Sangeet Natak Akademi Award.
Once again, bravo for your work. When you have a precise idea about the cost of your analyzer, please let me know. I shall be able to propose it to research institutions in Asian countries, and our own research institution, provided that it can afford it, might also acquire such an analyzer for our own work.
— Tran Van KHE, Director of Research, CNRS, Paris.
The equipment which Mr. E.J. Arnold and B. Bel propose to construct in the second stage of the research which they have explained to me seems to be of very great interest for the elucidation of the problems concerning scales, and intonation, as much from the point of view of their artistic and musicological use, as from the theory of acoustics.
— Iannis XENAKIS, Composer, Paris.